
A railway train is travelling on a curve of 750m radius at 30km/hr through to what angle it turns in 10 second.
Answer
497.4k+ views
Hint:
First of all we’ll use the distance formula which is Distance formula
Complete step by step solution:
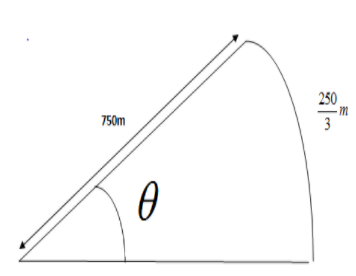
Given: Radius of the curve =750 m.
Speed at the railway train in travelling =30km/hr
Distance travelled by the train in 10 second;
Now, using Arc length formula;
The train will make an angle of
Note:
This is a classic problem connecting our real life to mathematics. In higher studies, the same question can be asked with a slight change. They’ll give a certain condition and say the train is running on the path which follows this condition. We have to find the path of the train. This kind of problem can be solved by locus concept.
First of all we’ll use the distance formula which is Distance formula
Complete step by step solution:

Given: Radius of the curve =750 m.
Speed at the railway train in travelling =30km/hr
Distance travelled by the train in 10 second;
Now, using Arc length formula;
The train will make an angle of
Note:
This is a classic problem connecting our real life to mathematics. In higher studies, the same question can be asked with a slight change. They’ll give a certain condition and say the train is running on the path which follows this condition. We have to find the path of the train. This kind of problem can be solved by locus concept.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




