Question

A regular polygon has angles of size 150 degrees each. How many sides does the polygon have?

Answer 1

Hint: We will look at the definition of a regular polygon. We will see some examples of regular polygons and also the properties of a regular polygon. We will find the measure of the exterior angle using the given measure of the interior angle. We will use the property of the exterior angles of a regular polygon to find the number of sides of the regular polygon.

Complete step by step answer:
A regular polygon is defined as a polygon that has all the sides of equal length and all the angles of the same measure. An equilateral triangle is a regular polygon that has three sides. A square is a regular polygon that has four sides.
In a regular polygon, the sum of an interior angle and its exterior angle is $180{}^\circ $. We will use this property to find the measure of the exterior angle of the regular polygon given in the question. Its interior angle has measure $150{}^\circ $. Therefore, the exterior angle has measure $180{}^\circ -150{}^\circ =30{}^\circ $.
Regular polygons have the property that the sum of all its exterior angles is $360{}^\circ $. Using this property, we can see that
$\text{number of sides}=\dfrac{\text{sum of exterior angles}}{\text{measure of one exterior angle}}$
Substituting the respective values, we get the following,
\[\begin{align}
  & \text{number of sides}=\dfrac{360~{}^\circ }{30{}^\circ } \\
 & \therefore \text{number of sides}=12 \\
\end{align}\]
Therefore, the number of sides of the regular polygon with interior angle $150{}^\circ $ is 12. This regular polygon looks like the following,

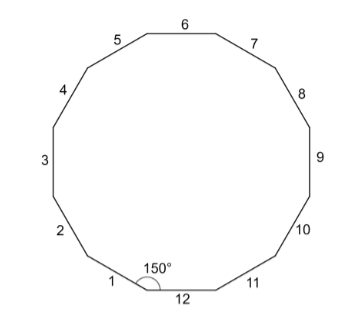
Note: The sum of the interior angles of this regular polygon will be $150{}^\circ \times 12=1800{}^\circ $. It is useful to know the relation between the interior angles, the exterior angles and the number of sides of a regular polygon. There is a formula to find the sum of interior angles of a polygon using the number of sides. This formula uses the fact that we can divide a regular polygon into triangles.