
A road roller takes
Answer
432k+ views
Hint: In the given question, we have to find the area of the road. In one revolution, the road roller will cover an area equal to its lateral surface. Since the road roller is in the shape of a cylinder, we can find the lateral surface area with the help of formula
Complete step by step solution:
In this given problem,
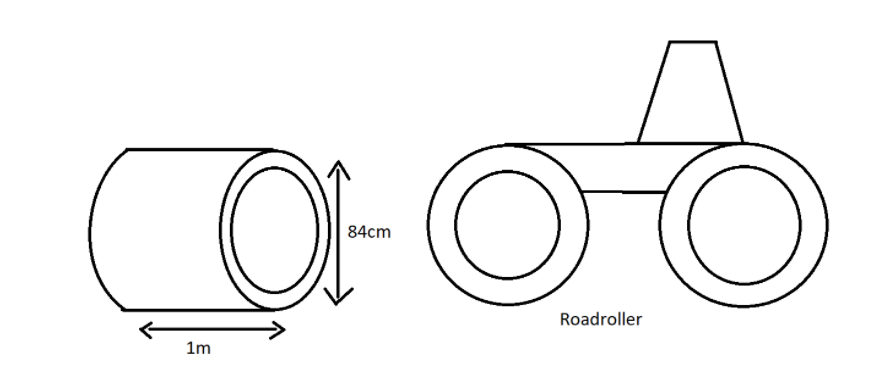
Road-roller is used to level the ground and its wheels are generally in the shape of a cylinder. It is shown in diagram as follows:
The formula for lateral surface area of cylinder is:
We are given that-
Therefore, Radius of the cylinder,
Substitute all values in the formula, the lateral surface area of road roller will be:
Now the area of the road will be total revolutions completed by the road-roller multiplied by the LSA of road-roller:
Thus, the area of the road is
So, the correct answer is “
Note: The key point in this question is to identify the formula and shape of the road roller.
A cylinder is one of the most common three-dimensional shapes, with two parallel circular bases separated by a space.
A curved surface connects the two circular bases at a fixed distance from the middle. The axis of the cylinder is a line segment that connects the centres of two circular bases. The height of the cylinder is the distance between the two circular bases. Example – LPG cylinder.
Complete step by step solution:
In this given problem,
Road-roller is used to level the ground and its wheels are generally in the shape of a cylinder. It is shown in diagram as follows:

The formula for lateral surface area of cylinder is:
We are given that-
Therefore, Radius of the cylinder,
Substitute all values in the formula, the lateral surface area of road roller will be:
Now the area of the road will be total revolutions completed by the road-roller multiplied by the LSA of road-roller:
Thus, the area of the road is
So, the correct answer is “
Note: The key point in this question is to identify the formula and shape of the road roller.
A cylinder is one of the most common three-dimensional shapes, with two parallel circular bases separated by a space.
A curved surface connects the two circular bases at a fixed distance from the middle. The axis of the cylinder is a line segment that connects the centres of two circular bases. The height of the cylinder is the distance between the two circular bases. Example – LPG cylinder.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




