
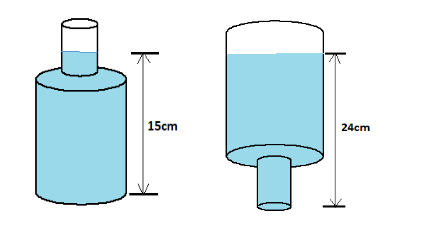
A sealed bottle containing some water is made up of two cylinders
A.
B.
C.
D.

Answer
438.3k+ views
Hint: In this problem, we need to convert this word problem into mathematical expression. Here, the sealed bottle containing some water made up of two cylinders of radius is given in the problem. When the bottle is placed upside down on the table the notice the height of the water is given then finally find the height of the bottle by using the volume of cylinder formula is
Where,
Complete step by step solution:
In the given problem,
Let the two cylinder be
Radius of
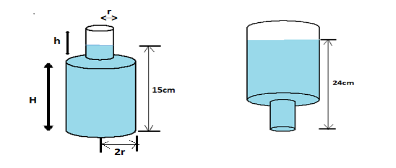
To find the height of the cylinder, we get
Total height
The formula for finding the volume of cylinder,
The first sealed bottle has two cylinder shape is equal to the second inverted cylinder, we get
Take out
By dividing on both sides by
By simplify the arithmetic operation, we get
To simplify in further step, we have
So, now we get
Total height,
Therefore, the height of the cylinder is
So, the correct answer is “
Note: We note that the cylinder shaped sealed bottle has filled with water.so, the height of water is mention and we have to find the total height of the cylinder by using the volume of the cylinder formula is
Where,
Complete step by step solution:
In the given problem,
Let the two cylinder be
Radius of

To find the height of the cylinder, we get
Total height
The formula for finding the volume of cylinder,
The first sealed bottle has two cylinder shape is equal to the second inverted cylinder, we get
Take out
By dividing on both sides by
By simplify the arithmetic operation, we get
To simplify in further step, we have
So, now we get
Total height,
Therefore, the height of the cylinder is
So, the correct answer is “
Note: We note that the cylinder shaped sealed bottle has filled with water.so, the height of water is mention and we have to find the total height of the cylinder by using the volume of the cylinder formula is
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




