
A tetrahedron has 4 faces and 6 edges. How many vertices does it have?
(a). 2
(b). 4
(c). 6
(d). 8
Answer
512.4k+ views
Hint: We have a tetrahedron that has four faces and 6 edges. We have to find the number of vertices. Use the Euler’s polyhedron formula,
Complete step-by-step answer:
According to the question, it is given that a tetrahedron has 4 faces and 6 edges. We are required to find the exact number of vertices of the tetrahedron.
We know that tetrahedron is a form of a polyhedron. So, we can apply Euler’s polyhedron formula here.
According to Euler’s polyhedron formula, we have
, where V is the number of vertices, e is the number of edges and F is the number of faces.
In a tetrahedron,
Number of faces = 4 ……………………(2)
Number of edges = 6 …………………..(3)
From equation (1), equation (2) and equation (3), we get
On solving, we get
Hence, the number of vertices is 4.
Therefore, option (B) is the correct one.
Note: One can also solve this question with the help of a figure of tetrahedron. We have the point E,F, and G in the vertical plane and point H coming out of the plane EFG.
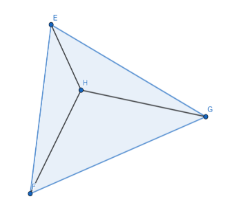
In this figure, we have plane EFG, EHF, EHG, and FHG as the face of the tetrahedron and EF, EG, EH, FG, FH, and GH as the edges of the tetrahedron. Points E, F, G, and H are the vertices of the tetrahedron.
Hence, the number of vertices is 4.
But don’t proceed with this approach because it is very difficult to imagine the figure if the given polyhedron is octahedron or something else complex.
Complete step-by-step answer:
According to the question, it is given that a tetrahedron has 4 faces and 6 edges. We are required to find the exact number of vertices of the tetrahedron.
We know that tetrahedron is a form of a polyhedron. So, we can apply Euler’s polyhedron formula here.
According to Euler’s polyhedron formula, we have
, where V is the number of vertices, e is the number of edges and F is the number of faces.
In a tetrahedron,
Number of faces = 4 ……………………(2)
Number of edges = 6 …………………..(3)
From equation (1), equation (2) and equation (3), we get
On solving, we get
Hence, the number of vertices is 4.
Therefore, option (B) is the correct one.
Note: One can also solve this question with the help of a figure of tetrahedron. We have the point E,F, and G in the vertical plane and point H coming out of the plane EFG.

In this figure, we have plane EFG, EHF, EHG, and FHG as the face of the tetrahedron and EF, EG, EH, FG, FH, and GH as the edges of the tetrahedron. Points E, F, G, and H are the vertices of the tetrahedron.
Hence, the number of vertices is 4.
But don’t proceed with this approach because it is very difficult to imagine the figure if the given polyhedron is octahedron or something else complex.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




