Question

A uniformly charged thin spherical shell of radius \[\] carries uniform surface charge density of $\sigma $ per unit area. It is made of two hemispherical shells, held together by pressing them with force $F$ (see figure). $F$ is proportional to:

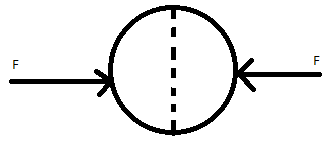
_{A. $\dfrac{1}{{{\varepsilon _ \circ }}}{\sigma ^2}{R^2}$
B. $\dfrac{1}{{{\varepsilon _ \circ }}}{\sigma ^2}R$
C. $\dfrac{1}{{{\varepsilon _ \circ }}}\dfrac{{{\sigma ^2}}}{R}$
D. $\dfrac{1}{{{\varepsilon _ \circ }}}\dfrac{{{\sigma ^2}}}{{{R^2}}}$}

Answer 1

Hint:
First let us see what pressure is:
The amount of force exerted per area is known as pressure.
So, force = pressure × area
We have to use this relation in this solution to find the force.

Complete step by step solution:
Now let us find the electrostatic pressure on the surface of a charged conductor-

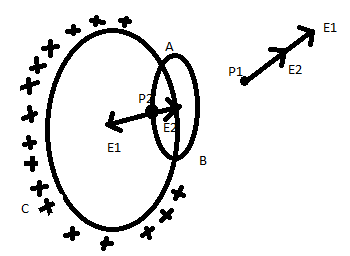
On its surface, the charge given to a conductor is evenly distributed. A repulsive force acts on the conductor through the charge on the rest part of the charge present on the conductor at the small element, and hence, a repulsive force acts on the conductor at each small element, and the overall force on the conductor’s surface is the amount of force vector acting on all the small elements.
It is why the charged conductor has pressure on the charged conducting surface outside.
Let the density of surface charge on the conductor’s surface be $\sigma $ . The points outside and within the conductor, be labelled as points ${P_1}$ and ${P_2}$ respectively.
The electric field outside the charged conducting surface is $\dfrac{\sigma }
{{{\varepsilon _ \circ }}}$ .
Thus, electric field at point ${P_1}$ is
${E_{P1}} = \dfrac{\sigma }
{{{\varepsilon _ \circ }}}$ ...... (i)

Electric field inside the conductor is zero. Thus, electric field at ${P_2}$ is-
${E_{P2}} = 0$ ...... (ii)

Now, we divide the conductor into two parts:
a. Element $AB$ whose surface is ds
b. The remaining part $ACB$

Let the electric field intensity near element $AB$ be ${E_1}$ and in the part $ACB$ be ${E_2}$
${E_{P1}} = {E_1} + {E_2}$ ...... (iii)

( ${E_1}$ and ${E_2}$ are in the same direction at ${P_1}$ )
${E_{P2}} = {E_1} - {E_2}$ ...... (iv)

( ${E_1}$ and ${E_2}$ are in the opposite direction at ${P_2}$ )
From equations (2) and (4), we get-
${E_1} - {E_2} = 0$
${E_1} = {E_2}$ ...... (v)

From equations (i), (iii) and (v), we get-
${E_2} = \dfrac{\sigma }
{{2{\varepsilon _ \circ }}}$

We take this as the pressure,
$P = \dfrac{\sigma }
{{2{\varepsilon _ \circ }}}$

Hence, force = pressure × area
$ = \dfrac{\sigma }
{{2{\varepsilon _ \circ }}} \times \pi {R^2}$

If we take $\dfrac{\pi } {2}$ as the constant, then
Force $\alpha \dfrac{1}
{{{\varepsilon _ \circ }}}{\sigma ^2}{R^2}$

Hence, option A is the answer.

Note:
Here we have to remember or establish the relationship for pressure. Only then we will be able to find the force for the given question.