
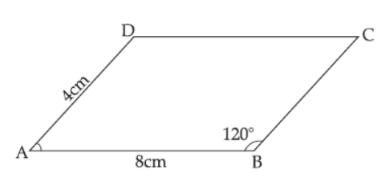
ABCD is a parallelogram with AB=8cm, AD=4cm,
(a) What is
(b) What is the perpendicular distance from D to AB?
(c) What is the area of ABCD?

Answer
519.9k+ views
Hint: Use the property of parallelogram that opposite angles of a parallelogram are equal. Then use the property that the sum of all angles of a quadrilateral is
Complete step-by-step answer:
We are given that ABCD is a parallelogram with AB=8cm, AD=4cm,
We need to find the following
(a)
(b) the perpendicular distance from D to AB
(c) the area of ABCD
Let us first solve (a).
We know that the opposite angles of a parallelogram are equal.
Using this,
And,
Also, we know that the sum of all angles of a quadrilateral is
Substituting the relations between the angles in this equation, we will get the following:
So,
Now, let us solve (b)
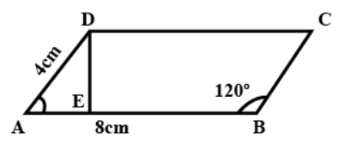
For this, draw DE as perpendicular from D to AB as shown in the figure.
We need to find the length of DE.
Now, we found in the previous part that
We also know that
So,
This gives us:
So, the perpendicular distance from D to AB is
Now, we will solve (c).
We need to find the area of ABCD.
We know that:
Area of parallelogram = Length of base
Here, we have length of base, AB = 8 cm
Height, DE =
Putting these values in the formula, we will get the following:
Area of ABCD = Length of AB
Area of ABCD =
So, Area of ABCD is
Note: You have to remember the various geometrical properties to solve this kind of question. For example, in this question too, you need to use the properties like opposite angles of a parallelogram are equal and that the sum of all angles of a quadrilateral is
Complete step-by-step answer:
We are given that ABCD is a parallelogram with AB=8cm, AD=4cm,
We need to find the following
(a)
(b) the perpendicular distance from D to AB
(c) the area of ABCD
Let us first solve (a).
We know that the opposite angles of a parallelogram are equal.
Using this,
And,
Also, we know that the sum of all angles of a quadrilateral is
Substituting the relations between the angles in this equation, we will get the following:
So,
Now, let us solve (b)
For this, draw DE as perpendicular from D to AB as shown in the figure.
We need to find the length of DE.

Now, we found in the previous part that
We also know that
So,
This gives us:
So, the perpendicular distance from D to AB is
Now, we will solve (c).
We need to find the area of ABCD.
We know that:
Area of parallelogram = Length of base
Here, we have length of base, AB = 8 cm
Height, DE =
Putting these values in the formula, we will get the following:
Area of ABCD = Length of AB
Area of ABCD =
So, Area of ABCD is
Note: You have to remember the various geometrical properties to solve this kind of question. For example, in this question too, you need to use the properties like opposite angles of a parallelogram are equal and that the sum of all angles of a quadrilateral is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




