Question

ABCD is a trapezium in which \[AB\parallel DC\] and \[AB = 2CD\]. The diagonals AC and BD meet at O. The ratio of areas of triangles AOB and COD is
A. \[1:4\]
B. \[1:2\]
C. $1:\sqrt 2 $
D. $4:1$

Answer 1

Hint: As given in the question that AB is parallel to CD, we take two triangles namely triangle AOB and triangle COD. Now, we have to prove that the triangles are similar. From the result obtained, we can easily say that the ratio of AB and CD is equal to the ratio of AO and CO and the ratio of OB and OD are equal. Now, we can easily find the ratio of 2CD and CD and by squaring the ratio we get the ratio of the area of both triangles.

Complete step-by-step solution:
The figure of the question based on data provided is,

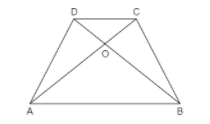
As given in the question that they AB is parallel to CD. Now we take triangle AOB and triangle COD to prove that both the triangles are similar.
So, we have to prove both the triangles $\Delta AOB$ and $\Delta COD$ are similar.
In $\Delta AOB$ and $\Delta COD$,
$ \Rightarrow \angle OAB = \angle OCD$ (Using alternate interior angles)
$ \Rightarrow \angle OBA = \angle ODC$ (Using alternate interior angles)
By AA similarity,
$\Delta AOB \sim \Delta COD$
We know that,
$\dfrac{{Area\,of\,\Delta AOB}}{{Area\,of\,\Delta COD}} = {\left( {\dfrac{{AB}}{{CD}}} \right)^2}$
Substitute $2CD$ in place of $AB$,
$ \Rightarrow \dfrac{{Area\,of\,\Delta AOB}}{{Area\,of\,\Delta COD}} = {\left( {\dfrac{{2CD}}{{CD}}} \right)^2}$
Cancel out the common factor and square the term,
$\therefore \dfrac{{Area\,of\,\Delta AOB}}{{Area\,of\,\Delta COD}} = 4$
Thus, the ratio of the area of triangle AOB and COD is $4:1$.

Hence, the option(D) is the correct answer.

Note: The key step for solving this problem is the proof of similarity of both the triangles. Once both the triangles are similar then by using the ratio of sides, we can easily evaluate the ratio of the area of the mentioned triangles. So, the knowledge of similar figures is required to solve this problem.