
An element with density $2.8gc{m^{ - 3}}$forms a FCC unit cell with edge length $4 \times {10^{ - 8}}cm$. Calculate the molar mass of the element? (Given ${N_A} = 6.022 \times {10^{23}}moles$ )
Answer
463.5k+ views
Hint: By using the Mass-density relationship, we can determine the value for molar mass. Volume of the unit cell will be equivalent to the volume of the cube. Mass of the unit cell will be equal to the molecular mass of one atom times the total number of atoms in the unit cell.
Formula used:
$\rho = \dfrac{{Z \times m}}{{{a^3} \times {N_A}}}$
where,$\rho = density,Z = Number\,of\,atoms\,in\,a\,unit\,cell,m = molar\,mass,{N_A} = Avogadro\,Number,a = unit\,length$
Complete step by step solution:
Let us understand the terms we will be using:
FCC refers to Face centered Cubic lattice. Refer to the diagram below.
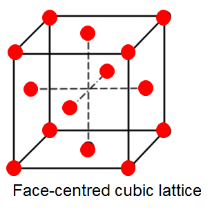
Here, we have $8$ atoms on the corners which are being shared with $8$ unit cells and $6$ atoms on the faces which are being shared with $2$ unit cells.
Therefore One unit cell will have
Number of atoms in one FCC unit cell $(Z) = \dfrac{1}{8} \times 8 + \dfrac{1}{2} \times 6 \Rightarrow 4$
$Z = 4$
Let us understand the formulae we will be using:
We known that Density is the ratio of Mass to Volume and can be written as
$Density = \dfrac{{Mass}}{{Volume}}$
For a cube, the volume can be modified as
$Volume = {(unit\,\,length)^3}$Since, all sides are equal in a cube.
Mass can be given as $Mass\,of\,1\,atom = \dfrac{{Molar\,Mass}}{{Avogadro's\,Number}}$
Number of atoms in a unit cell is given by Z and hence mass of the atoms in a unit cell can be given by $Mass\,of\,atoms\,in\,a\,unit\,cell = \dfrac{{Z \times M}}{{{N_A}}}$
Substituting the value of Mass of atom in a unit cell, in the above equation, we get,
\[Density = \dfrac{{Z \times Molar\,Mass}}{{{{(unit\,length)}^3} \times {N_A}}}\]
which can also be written as $\rho = \dfrac{{Z \times m}}{{{a^3} \times {N_A}}}$
$\rho = density,Z = Number\,of\,atoms\,in\,a\,unit\,cell,m = molar\,mass,{N_A} = Avogadro\,Number,a = unit\,length$
From the question, we know the values of $\rho = 2.8gc{m^{ - 3}},a = 4 \times {10^{ - 8}}$ and we have calculates that for FCC the value of $Z = 4$ .
Substituting these values we get:
$mass = \dfrac{{2.8 \times {{(4 \times {{10}^{ - 8}})}^3} \times 6.022 \times {{10}^{23}}}}{4}$
$mass = 26.97gmo{l^-1 }$
Hence the $molar\,mass = 26.97gmo{l^-1 }$.
Note:
-The Value for $Z$ changes with different Lattices. The highest Value of $Z$ is $4$ which is in the case of Face centered Cubic lattice and the lowest possible value of $Z$ is $1$ which is in case of Simple cubic lattice.
-Face centered Lattice can be formed in Cubic close Packing and Hexagonal close packing, Although the Value for $Z$ does not change, but the formula to calculate the volume of the hexagon will be different for the volume of a cube.
Formula used:
$\rho = \dfrac{{Z \times m}}{{{a^3} \times {N_A}}}$
where,$\rho = density,Z = Number\,of\,atoms\,in\,a\,unit\,cell,m = molar\,mass,{N_A} = Avogadro\,Number,a = unit\,length$
Complete step by step solution:
Let us understand the terms we will be using:
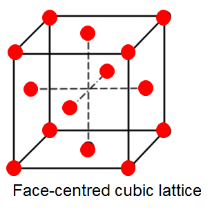
FCC refers to Face centered Cubic lattice. Refer to the diagram below.

Here, we have $8$ atoms on the corners which are being shared with $8$ unit cells and $6$ atoms on the faces which are being shared with $2$ unit cells.
Therefore One unit cell will have
Number of atoms in one FCC unit cell $(Z) = \dfrac{1}{8} \times 8 + \dfrac{1}{2} \times 6 \Rightarrow 4$
$Z = 4$
Let us understand the formulae we will be using:
We known that Density is the ratio of Mass to Volume and can be written as
$Density = \dfrac{{Mass}}{{Volume}}$
For a cube, the volume can be modified as

$Volume = {(unit\,\,length)^3}$Since, all sides are equal in a cube.
Mass can be given as $Mass\,of\,1\,atom = \dfrac{{Molar\,Mass}}{{Avogadro's\,Number}}$
Number of atoms in a unit cell is given by Z and hence mass of the atoms in a unit cell can be given by $Mass\,of\,atoms\,in\,a\,unit\,cell = \dfrac{{Z \times M}}{{{N_A}}}$
Substituting the value of Mass of atom in a unit cell, in the above equation, we get,
\[Density = \dfrac{{Z \times Molar\,Mass}}{{{{(unit\,length)}^3} \times {N_A}}}\]
which can also be written as $\rho = \dfrac{{Z \times m}}{{{a^3} \times {N_A}}}$
$\rho = density,Z = Number\,of\,atoms\,in\,a\,unit\,cell,m = molar\,mass,{N_A} = Avogadro\,Number,a = unit\,length$
From the question, we know the values of $\rho = 2.8gc{m^{ - 3}},a = 4 \times {10^{ - 8}}$ and we have calculates that for FCC the value of $Z = 4$ .
Substituting these values we get:
$mass = \dfrac{{2.8 \times {{(4 \times {{10}^{ - 8}})}^3} \times 6.022 \times {{10}^{23}}}}{4}$
$mass = 26.97gmo{l^-1 }$
Hence the $molar\,mass = 26.97gmo{l^-1 }$.
Note:
-The Value for $Z$ changes with different Lattices. The highest Value of $Z$ is $4$ which is in the case of Face centered Cubic lattice and the lowest possible value of $Z$ is $1$ which is in case of Simple cubic lattice.
-Face centered Lattice can be formed in Cubic close Packing and Hexagonal close packing, Although the Value for $Z$ does not change, but the formula to calculate the volume of the hexagon will be different for the volume of a cube.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

What is a transformer Explain the principle construction class 12 physics CBSE

What are the major means of transport Explain each class 12 social science CBSE




