
An equilateral triangle has an altitude of
Answer
397.8k+ views
Hint: We will use the concepts in geometry such as triangles and their properties to solve the given problem.
We will define different types of triangles and their properties by illustrating them. We will use Pythagoras theorem too while solving this problem.
In mathematics, a triangle is a polygon (a closed figure) with three sides.
Equilateral triangle – A triangle with all sides equal.
In a triangle, a perpendicular drawn from a point to the side opposite to that point is called the altitude of a triangle.
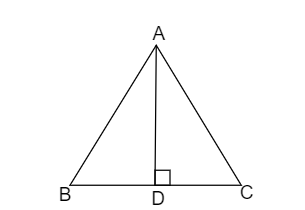
Here, the line
In an equilateral triangle, all sides are equal and that implies that all angles are equal to
Complete step by step solution:
Consider the figure.
Let the side of an equilateral triangle be ‘
From the triangle,
By applying Pythagoras theorem in
So, we get,
And this is the relation between altitude of an equilateral triangle and the side of an equilateral triangle.
So, now, it is given that, the altitude of an equilateral triangle is
Substituting this in the above relation, we get,
So, side of this equilateral triangle is
And we all know that, perimeter of an equilateral triangle of length
Therefore, the perimeter of this equilateral triangle is
Note:
Other two types of triangles based on lengths of sides are:
(1) Scalene triangle – A triangle with all three sides of different lengths.

(2) Isosceles triangle – A triangle with two sides equal.
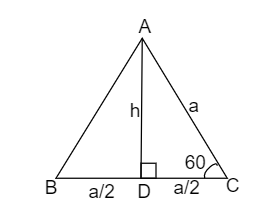
> We found the relation between altitude and side by applying Pythagoras theorem. But instead, we can also do it in another way.
In triangle
And we know that all angles of an equilateral triangle are
And we know that,
And using this, we can solve our required problem.
We will define different types of triangles and their properties by illustrating them. We will use Pythagoras theorem too while solving this problem.
In mathematics, a triangle is a polygon (a closed figure) with three sides.
Equilateral triangle – A triangle with all sides equal.

In a triangle, a perpendicular drawn from a point to the side opposite to that point is called the altitude of a triangle.

Here, the line
In an equilateral triangle, all sides are equal and that implies that all angles are equal to
Complete step by step solution:
Consider the figure.

Let the side of an equilateral triangle be ‘
From the triangle,
By applying Pythagoras theorem in
So, we get,
And this is the relation between altitude of an equilateral triangle and the side of an equilateral triangle.
So, now, it is given that, the altitude of an equilateral triangle is
Substituting this in the above relation, we get,
So, side of this equilateral triangle is
And we all know that, perimeter of an equilateral triangle of length
Therefore, the perimeter of this equilateral triangle is
Note:
Other two types of triangles based on lengths of sides are:
(1) Scalene triangle – A triangle with all three sides of different lengths.

(2) Isosceles triangle – A triangle with two sides equal.

> We found the relation between altitude and side by applying Pythagoras theorem. But instead, we can also do it in another way.
In triangle
And we know that all angles of an equilateral triangle are
And we know that,
And using this, we can solve our required problem.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE




