
What are conjugate foci? Deduce the following expression between the focal length
Answer
504.9k+ views
Hint: Conjugate foci are points such that if we keep an object at one point, we get the rays focused on the other point and vice-versa. The mirror formula is derived by considering similar triangles in the ray diagram.
Complete step-by-step solution -
We know that light rays from a point A on the principal axis, after refraction from the lens, focus at another single point B on the axis.
Also, if we interchange these points and keep the source at B, the rays would focus at A. All such pairs of interchangeable points that are related as Source and image are called conjugate foci.
It is called foci because light from one such point focuses on the other.
Let us imagine a mirror, as shown in figure, which forms a real image of a source. Let us call the distance of the object from the pole
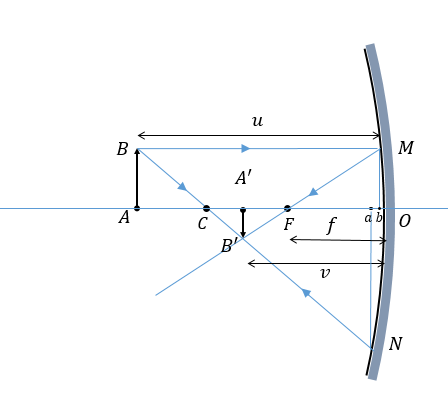
Let's draw two perpendiculars - from points M and N. Let these lines meet the principal axis at
To find the relation between v and u, let us consider the triangles
we see that
So we can say that
Now, since the corresponding parts of two similar triangles have the same ratio:
Now let us consider triangles
we see that
So we can say that
Now, since the corresponding parts of two similar triangles have the same ratio:
But
Let us compare (1) and (2)
Now we can substitute the values of
but we should apply sign conventions while measuring distances. All distances measured to the left are negative.
So,
So we can substitute these values into equation (3) to get:
Since
We can divide this equation by
Thus,
Note: There are infinitely many conjugate foci for mirrors and lenses. The centre of curvature of a mirror is a special point for which the image and object positions coincide. Also note that the same derivation can also be done for convex mirrors. proper sign conventions have to be used for u, v and f.
Complete step-by-step solution -
We know that light rays from a point A on the principal axis, after refraction from the lens, focus at another single point B on the axis.
Also, if we interchange these points and keep the source at B, the rays would focus at A. All such pairs of interchangeable points that are related as Source and image are called conjugate foci.
It is called foci because light from one such point focuses on the other.
Let us imagine a mirror, as shown in figure, which forms a real image of a source. Let us call the distance of the object from the pole

Let's draw two perpendiculars - from points M and N. Let these lines meet the principal axis at
To find the relation between v and u, let us consider the triangles
we see that
So we can say that
Now, since the corresponding parts of two similar triangles have the same ratio:
Now let us consider triangles
we see that
So we can say that
Now, since the corresponding parts of two similar triangles have the same ratio:
But
Let us compare (1) and (2)
Now we can substitute the values of
but we should apply sign conventions while measuring distances. All distances measured to the left are negative.
So,
So we can substitute these values into equation (3) to get:
Since
We can divide this equation by
Thus,
Note: There are infinitely many conjugate foci for mirrors and lenses. The centre of curvature of a mirror is a special point for which the image and object positions coincide. Also note that the same derivation can also be done for convex mirrors. proper sign conventions have to be used for u, v and f.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




