
How are the time period T and the frequency f of a simple pendulum related to each other?
Answer
461.1k+ views
Hint: The relation between the frequency and the time period can be derived for any motion in a similar method. The time period and the frequency involved in the simple pendulum is related to each other in the same way as it is related in any other case.
Complete step-by-step solution
To know the relation between the frequency and the time period of a particular motion, we should initially know what the two parameters actually mean. Let us consider a simple pendulum, which undergoes a simple harmonic motion.
Let us define the two quantities initially. The time period is defined as the time taken by the pendulum bob to come back to its mean or equilibrium position after a complete to-and-fro oscillation. In the figure below, it is the time taken for the bob to start from O, then to A, then return to O, then move to B and return back to O. Its SI unit is seconds as it denotes a time.
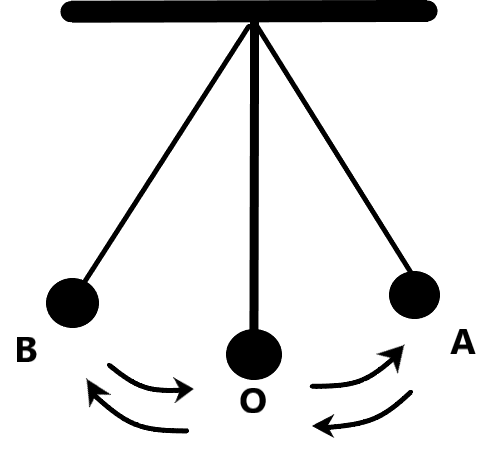
The frequency is defined as the number of oscillations that the simple pendulum can undergo in a unit of time. Its SI unit is Hertz which is more clearly the inverse of a second. From the information, we understand that the frequency is the reciprocal of the time period.
i.e.,
\[f=\dfrac{1}{T}\]
This is the required relationship between the time period T and the frequency f in a simple pendulum.
Note: We know that the quantities time period T and the frequency f in a simple harmonic motion remains constant. In the case of a simple pendulum, the time period and the frequency are dependent on the length of the pendulum string or the mass of the system.
Complete step-by-step solution
To know the relation between the frequency and the time period of a particular motion, we should initially know what the two parameters actually mean. Let us consider a simple pendulum, which undergoes a simple harmonic motion.
Let us define the two quantities initially. The time period is defined as the time taken by the pendulum bob to come back to its mean or equilibrium position after a complete to-and-fro oscillation. In the figure below, it is the time taken for the bob to start from O, then to A, then return to O, then move to B and return back to O. Its SI unit is seconds as it denotes a time.

The frequency is defined as the number of oscillations that the simple pendulum can undergo in a unit of time. Its SI unit is Hertz which is more clearly the inverse of a second. From the information, we understand that the frequency is the reciprocal of the time period.
i.e.,
\[f=\dfrac{1}{T}\]
This is the required relationship between the time period T and the frequency f in a simple pendulum.
Note: We know that the quantities time period T and the frequency f in a simple harmonic motion remains constant. In the case of a simple pendulum, the time period and the frequency are dependent on the length of the pendulum string or the mass of the system.
Recently Updated Pages
Glucose when reduced with HI and red Phosphorus gives class 11 chemistry CBSE

The highest possible oxidation states of Uranium and class 11 chemistry CBSE

Find the value of x if the mode of the following data class 11 maths CBSE

Which of the following can be used in the Friedel Crafts class 11 chemistry CBSE

A sphere of mass 40 kg is attracted by a second sphere class 11 physics CBSE

Statement I Reactivity of aluminium decreases when class 11 chemistry CBSE

Trending doubts
The correct order of melting point of 14th group elements class 11 chemistry CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

Define least count of vernier callipers How do you class 11 physics CBSE

The combining capacity of an element is known as i class 11 chemistry CBSE

Find the image of the point 38 about the line x+3y class 11 maths CBSE




