Question

What is the area of a regular hexagon inscribed in a circle of radius r ? \[\]
A. $ 2\sqrt{3}{{r}^{2}}\text{ sq}\text{.units } $ \[\]
B. $ \dfrac{3\sqrt{3}}{2}{{r}^{2}}\text{ sq}\text{.units } $ \[\]
C. $ \dfrac{2}{\sqrt{3}}{{r}^{2}}\text{ sq}\text{.units } $ \[\]
D. $ \dfrac{\sqrt{3}}{2}{{r}^{2}}\text{ sq}\text{.units } $ \[\]

Answer 1

Hint: We recall the shape of a regular hexagon and circle. We see that in a hexagon inscribed in a circle the length of the line segment joining the center of the regular hexagon (as well as the circle) is equal to the radius $ r $. We find the area of the regular hexagon as 6 times the area of an equilateral triangle formed by joining diametrically opposite vertices. Which means $ 6\times \dfrac{3\sqrt{3}}{4}{{a}^{2}} $ .\[\]

Complete step by step answer:
We know that we call a polygon regular when the lengths of its sides are equal. The longest diagonals of a regular polygon join diametrically opposite vertices, are of equal length, bisect the interior angle at subtended at the vertices and coincide at a point. The point is called center of the regular polygon. \[\]
The regular hexagon is a polygon with 6 sides and 6 vertices. A regular polygon that is inscribed in a circle will have all of the vertices on the circle and the centre of the circle will be center of the regular polygon.. We draw the regular polygon with ABCDEF with centre G below. \[\]

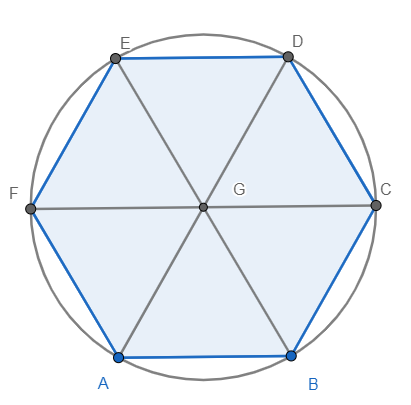
Let us consider the triangle AGB in the above figure. We measure of each angle in regular polygon is $ {{120}^{\circ }} $ . Since the longest diagonals AG and BG bisect $ \angle FAB={{120}^{\circ }},\angle DAC={{120}^{\circ }} $ respectively we have
\[\Rightarrow \angle GAB=\angle GBA=\dfrac{{{120}^{\circ }}}{2}={{60}^{\circ }}\]
Since sum of the angles in triangle is $ {{180}^{\circ }} $ , we have
\[\begin{align}
  & \Rightarrow \angle AGB={{180}^{\circ }}-\left( \angle GAB+\angle GBA \right) \\
 & \Rightarrow \angle AGB={{180}^{\circ }}-\left( {{60}^{\circ }}+{{60}^{\circ }} \right) \\
 & \Rightarrow \angle AGB={{180}^{\circ }}-{{120}^{\circ }}={{60}^{\circ }} \\
\end{align}\]
Since we have $ \angle GAB=\angle GBA=\angle AGB={{60}^{\circ }} $ , the triangle AGB is an equilateral triangle. Similarly we can prove that AGF, FGE, EGD, DGC, BGC are equilateral triangles.
We also see that the radius of the circle is half of the diagonal and is equal to the sides of the hexagon since all sides of an equilateral triangle are equal. So we have are of triangle equilateral triangle AGB is
\[{{A}_{t}}=\dfrac{\sqrt{3}}{4}{{\left( AB \right)}^{2}}=\dfrac{\sqrt{3}}{4}{{r}^{2}}\]
The area of the hexagon $ {{A}_{h}} $ is sum areas of 6 equilateral triangles which emans
\[{{A}_{h}}=6\times \dfrac{\sqrt{3}}{4}{{r}^{2}}=\dfrac{3\sqrt{3}}{2}{{r}^{2}}\]
So the correct option is B.\[\]
Note:
We know that the measure of an interior angle of a regular polygon with $ n $ sides is given by $ \dfrac{n-2}{n}\times {{180}^{\circ }} $ . The circle inscribing the hexagon is also called the circumradius of the hexagon. We find can also find the area $ {{A}_{h}} $ with apothem (perpendicular dropped from centre of regular polygon on a side ) $ p $ and side $ a $ as $ {{A}_{h}}=\dfrac{1}{2}ap $ .