
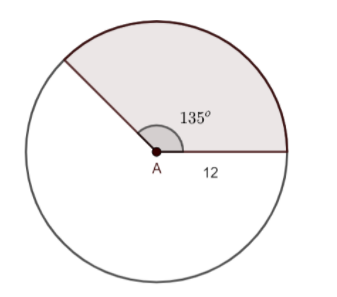
What is the area of the sector?
A.
B.
C.
D.

Answer
408.3k+ views
Hint: Here we have to find the area of the sector given. Firstly we will write down all the information like the angle of the sector, its radius or diameter which of them is given in the diagram. Then we will write the formula used for finding the area of a sector. Finally we will substitute all the values in the formula and solve it to get the desired answer.
Complete step-by-step answer:
The diagram of the circle and the sector in it is given as follows:
The information given in the diagram is as follows:
Angle of sector
Radius of the circle
The area of the sector is calculated by the below formula:
On substitute the values from equation (1) and (2) in above formula we get,
Put
Simplifying them completely we get,
Approximately we can write the value as,
So, the correct answer is “Option A”.
Note: A sector is a pie-shaped part of a circle which is made up of the arc of the circle along with the two radii of the circle. It looks like a pizza slice or as the name suggests like a pie. In it the simplest shape in geometry it has various parts such as diameter, radius, circumference, sector and segment. The circle is divided into two sectors the bigger part is known as the major sector while the smaller part is known as the minor sector. A special case when a circle is divided into two equal parts is known as a semi-circle.
Complete step-by-step answer:
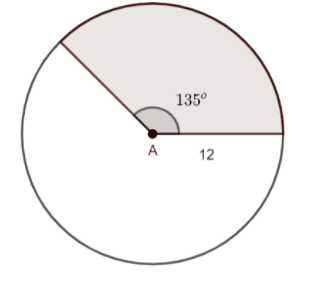
The diagram of the circle and the sector in it is given as follows:

The information given in the diagram is as follows:
Angle of sector
Radius of the circle
The area of the sector is calculated by the below formula:
On substitute the values from equation (1) and (2) in above formula we get,
Put
Simplifying them completely we get,
Approximately we can write the value as,
So, the correct answer is “Option A”.
Note: A sector is a pie-shaped part of a circle which is made up of the arc of the circle along with the two radii of the circle. It looks like a pizza slice or as the name suggests like a pie. In it the simplest shape in geometry it has various parts such as diameter, radius, circumference, sector and segment. The circle is divided into two sectors the bigger part is known as the major sector while the smaller part is known as the minor sector. A special case when a circle is divided into two equal parts is known as a semi-circle.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE





