Question

At the centre of a cubical box $ + Q$ charge is placed. The value of total flux that is coming out of each face is:
(A) $Q/{ \in _0}$
(B) $Q/3{ \in _0}$
(C) $Q/4{ \in _0}$
(D) $Q/6{ \in _0}$

Answer 1

Hint:Find whether the given surface is Gaussian surface or not. If it is a Gaussian surface apply the gauss formula and substitute the number of faces that it encloses to find the electric flux that comes out of each face of the given closed surface.

Useful formula:
The Gaussian law is given as
$f = \dfrac{Q}{{{ \in _0}}}$
Where $f$ is the total electric flux, $Q$ is the charge enclosed by the surface and ${ \in _0}$ is the permittivity of the free space.

Complete step by step solution:
It is given that the charge of $ + Q$ is placed at the centre of the box. The Gauss’s law provides the information about the distribution of the electric charges for the closed surface. In the closed surface, the electric flux is directly proportional to the electric charges enclosed in the surface.

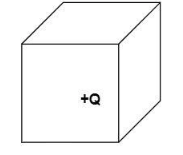
In the given question, the cubical surface encloses the charge $ + Q$ . Since the cube is the closed surface with the six faces, the gauss law can be applied to find the electric flux of it.

By using the formula of the Gaussian law,

$f = \dfrac{Q}{{{ \in _0}}}$

Since the cube contains the six faces, the permittivity of the free space is multiplied by six to find the flux from each face.

$f = \dfrac{Q}{{6{ \in _0}}}$

Hence the electric flux that is coming out of each flux is obtained as $\dfrac{Q}{{6{ \in _0}}}$

Thus the option (D) is correct.

Note:The Gauss law is used for the determination of electric field over the closed surface and the charge distribution in it. Remember that if the charge $Q$ is placed outside of the cube, there will be no electric flux on the closed surface. Hence the charge on the surface alone can contribute to the electric flux.