
Calculate the perimeter and area of a quadrant of a circle whose radii are
i) 98
ii) 70
iii) 42
iv) 28
Answer
523.5k+ views
1 likes
Hint: Perimeter can be defined as the total length of the boundary of a geometrical figure. Area can be defined as the space occupied by a flat shape or the surface of an object. So, using this definition we can easily solve our problem.
Complete step-by-step answer:
If a circle is drawn from the origin, then the quadrant of a circle can be defined as a portion of the circle which lies between the positive x-axis and positive y-axis.
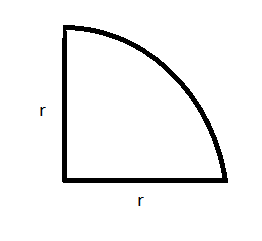
In general, the perimeter of the quadrant of circle
The area of quadrant of a circle
i) Perimeter of quadrant of a circle of radius 98 cm
Area of quadrant of circle of radius 98 cm
ii) Perimeter of quadrant of a circle of radius 70 cm
Area of quadrant of circle of radius 70 cm
iii) Perimeter of quadrant of a circle of radius 42 cm
Area of quadrant of circle of radius 42 cm
iv) Perimeter of quadrant of a circle of radius 28 cm
Area of quadrant of circle of radius 28 cm
Note: The key step for solving this problem is the knowledge of area and perimeter of a geometrical figure. In this case, the given figure is a quadrant of a circle. So, on constructing the figure we obtain the area and parameter in generalized form. After putting values in the formula, we get the desired result.
Complete step-by-step answer:
If a circle is drawn from the origin, then the quadrant of a circle can be defined as a portion of the circle which lies between the positive x-axis and positive y-axis.
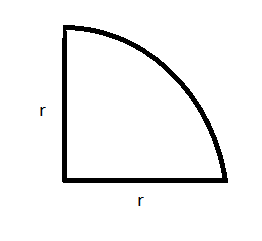
In general, the perimeter of the quadrant of circle
The area of quadrant of a circle
i) Perimeter of quadrant of a circle of radius 98 cm
Area of quadrant of circle of radius 98 cm
ii) Perimeter of quadrant of a circle of radius 70 cm
Area of quadrant of circle of radius 70 cm
iii) Perimeter of quadrant of a circle of radius 42 cm
Area of quadrant of circle of radius 42 cm
iv) Perimeter of quadrant of a circle of radius 28 cm
Area of quadrant of circle of radius 28 cm
Note: The key step for solving this problem is the knowledge of area and perimeter of a geometrical figure. In this case, the given figure is a quadrant of a circle. So, on constructing the figure we obtain the area and parameter in generalized form. After putting values in the formula, we get the desired result.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE




