Question

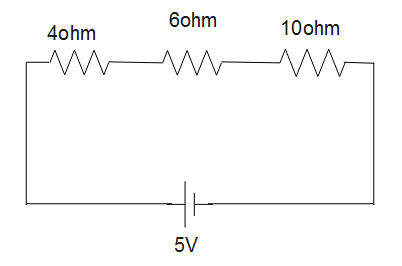
Calculate the potential difference across the resistor in the given circuit:

Answer 1

Hint: In this question we will directly use Ohm’s law, which gives the relation between the current, voltage, and resistance. Further, by substituting the given values, we will get the required result. Also, we will discuss the basics of an electrical circuit for our better understanding.
Formula used:
$\eqalign{& I \propto V \cr
& I = \dfrac{V}{R} \cr} $

Complete step-by-step solution
As we know, during steady-state, the inductor acts as a closed circuit, whereas the capacitor acts as an open circuit.
Also, as we know that according to the ohm’s law on constant temperature the current I pass through the conductor is directly proportional to the potential difference V across its ends.
$\eqalign{& I \propto V \cr
  & \Rightarrow I = \dfrac{V}{R} \cr} $
Here R is the equivalent resistance of circuit which is R=${R_1}+{R_2}+{R_3}$=$4+6+10= 20$
Now, by substituting the given values in above equation we get
$I = \dfrac{5}{{20}} = \dfrac{1}{4}A$
Now, we find the potential drop across resistor (1):
$V = IR$
Substituting the given values, we get:
$\eqalign{& V = \dfrac{1}{4} \times 4 \cr
  & \Rightarrow V = 1V \cr} $
Similarly, we find the potential drop across resistor (2):
$V = IR$
Substituting the given values, we get:
$\eqalign{& V = \dfrac{1}{4} \times 6 \cr
  & \Rightarrow V = \dfrac{3}{2}V \cr} $
Similarly, we find the potential drop across resistor (3):
$V = IR$
Substituting the given values, we get:
$\eqalign{& V = \dfrac{1}{4} \times 10 \cr
  & \Rightarrow V = \dfrac{5}{2}V \cr} $
Therefore, we get the required answer, which gives us the potential difference across the given three resistors.
Additional information: We should know that in a series circuit, the output current of the first resistor flows into the input of the second resistor; so, the current is the same in each resistor whereas In a parallel circuit, all of the resistors are on connected together on one side and all the leads on the other side are connected together.
In a circuit, if the resistance is constant over a range of voltage, then $I = \dfrac{V}{R}$, which can be used to predict the behavior of the material. This involves DC current and voltage, it is the same for the resistors. Further, a material obeys Ohm's law or does not obey; the resistance of the material can be described in terms of its bulk. The resistivity, and the resistance both, are temperature-dependent. Over certain ranges of temperature, this temperature dependence can be predicted from resistance.

Note: There are limitations to Ohm’s law. They are valid only for conductors not for all materials. We should also note that resistance is inversely proportional to the flow of current. The unit of resistance is the ohm, named after the scientist. The internal resistance of a circuit refers to the opposition to the flow currently offered by the cells and batteries themselves thereby, resulting in the generation of heat.