Question

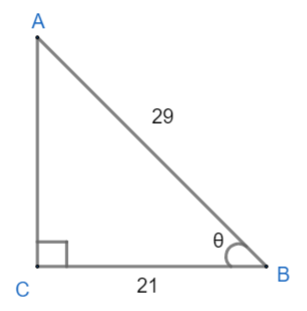
Consider triangle ABC, right angled at C in which AB = 29 units, BC = 21 units and $\angle ABC=\theta $ (see fig.) Determine the value of ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $.

Answer 1

Hint: In this question, we are given a right angled triangle along with measurements of base and hypotenuse of the triangle. We need to find the value of ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $. For this, we will first find the value of the perpendicular of the right angled triangle, using Pythagoras theorem. Pythagora's theorem states that, the sum of the squares of the perpendicular and the base of the triangle is equal to the square of the hypotenuse. After that, we will find the value of $\cos \theta \text{ and }\sin \theta $ and use them to find the value of ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $.
We will use $\cos \theta =\dfrac{\text{Side adjacent to angle }\theta }{\text{Hypotenuse}}=\dfrac{\text{Base}}{\text{Hypotenuse}}$ and
$\sin \theta =\dfrac{\text{Side opposite to angle }\theta }{\text{Hypotenuse}}=\dfrac{\text{Perpendicular}}{\text{Hypotenuse}}$.

Complete step-by-step answer:
Here we are given $\Delta ABC$ with AB = 29 and BC = 21. Since, $\Delta ABC=\theta $ so according to the diagram, base = 21 and hypotenuse = 29.

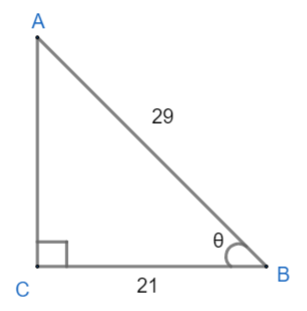
Now, let us first find the value of AC using Pythagoras theorem.
According to the Pythagoras theorem, the sum of the squares of the perpendicular and the base of the triangle is equal to the square of the hypotenuse. Hence,
\[{{\left( \text{Hypotenuse} \right)}^{2}}={{\left( \text{Perpendicular} \right)}^{2}}+{{\left( \text{Base} \right)}^{2}}\]
According to the diagram,
\[\begin{align}
  & {{\left( \text{AB} \right)}^{2}}={{\left( \text{AC} \right)}^{2}}+{{\left( \text{BC} \right)}^{2}} \\
 & \Rightarrow A{{C}^{2}}=A{{B}^{2}}-B{{C}^{2}} \\
\end{align}\]
Putting values of AB and BC we get:
\[\Rightarrow A{{C}^{2}}={{\left( 29 \right)}^{2}}-{{\left( 21 \right)}^{2}}\]
Let us find values of ${{\left( 29 \right)}^{2}}-{{\left( 21 \right)}^{2}}$ using the property that ${{a}^{2}}-{{b}^{2}}=\left( a+b \right)\left( a-b \right)$. Hence, we get:
\[\begin{align}
  & \Rightarrow A{{C}^{2}}=\left( 29+21 \right)\left( 29-21 \right) \\
 & \Rightarrow A{{C}^{2}}=\left( 50 \right)\left( 8 \right) \\
 & \Rightarrow A{{C}^{2}}=400 \\
\end{align}\]
Taking square root both sides, we get:
\[\Rightarrow AC=\sqrt{400}\]
Now, we know that, $20\times 20=400$ we get:
\[\Rightarrow AC=\sqrt{20\times 20}=20\]
So we get perpendicular = 20.
Now, let us find the value of $\cos \theta \text{ and }\sin \theta $.
As we know, $\cos \theta =\dfrac{\text{Side adjacent to angle }\theta }{\text{Hypotenuse}}=\dfrac{\text{Base}}{\text{Hypotenuse}}$.
Hence, $\cos \theta =\dfrac{21}{\text{29}}$.
As we know, $\sin \theta =\dfrac{\text{Side opposite to angle }\theta }{\text{Hypotenuse}}=\dfrac{\text{Perpendicular}}{\text{Hypotenuse}}$.
Hence, $\sin \theta =\dfrac{20}{\text{29}}$.
Now we need to find out the value of ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $.
So putting values of $\cos \theta \text{ and }\sin \theta $ in ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $ we get:
\[\begin{align}
  & \Rightarrow {{\left( \dfrac{21}{29} \right)}^{2}}+{{\left( \dfrac{20}{29} \right)}^{2}} \\
 & \Rightarrow \dfrac{{{\left( 21 \right)}^{2}}+{{\left( 20 \right)}^{2}}}{{{\left( 29 \right)}^{2}}} \\
 & \Rightarrow \dfrac{441+400}{841} \\
 & \Rightarrow \dfrac{841}{841} \\
 & \Rightarrow 1 \\
\end{align}\]
Hence the value of ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $ is 1.

Note: Students should take care while finding the value of perpendicular. Students can get confused between base and perpendicular while applying to the formula of $\cos \theta \text{ and }\sin \theta $. They can check their answer knowing that ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta $ is always 1 because $\cos \theta =\dfrac{B}{H}\text{ and }\sin \theta =\dfrac{P}{H}\Rightarrow {{\cos }^{2}}\theta +{{\sin }^{2}}\theta ={{\left( \dfrac{B}{H} \right)}^{2}}+{{\left( \dfrac{P}{H} \right)}^{2}}\Rightarrow {{\cos }^{2}}\theta +{{\sin }^{2}}\theta =\dfrac{{{B}^{2}}+{{P}^{2}}}{{{H}^{2}}}$. By Pythagoras theorem, ${{B}^{2}}+{{P}^{2}}={{H}^{2}}$ so ${{\cos }^{2}}\theta +{{\sin }^{2}}\theta =\dfrac{{{H}^{2}}}{{{H}^{2}}}=1$. Here, B is base, P is perpendicular and H is hypotenuse.