
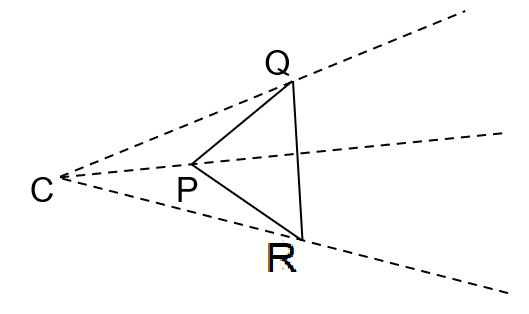
Construct a dilation, with scale factor 2, of a triangle using only a ruler and compasses.

Answer
477.6k+ views
1 likes
Hint: We use the centre of dilatation to join the given triangle. Then we use two processes of reduction and enlargement to find the required triangles. The conditions to do the dilation is
Complete step-by-step solution:
We follow some steps for the dilation process to dilate
Step: 1
We connect the center of dilation C to each vertex and extend those lines.
Step: 2
Then we place the point of the compass on the center of dilation C and the pencil on vertex Q to measure the distance.
Step: 3
Now, without changing the size of the compass, we will move the point of the compass to vertex Q, and make a mark on the line of extended CQ.
Step: 4
We now repeat the process for each of the other vertices of the triangle. Let’s assume the new vertices are
Step: 5
We connect the points
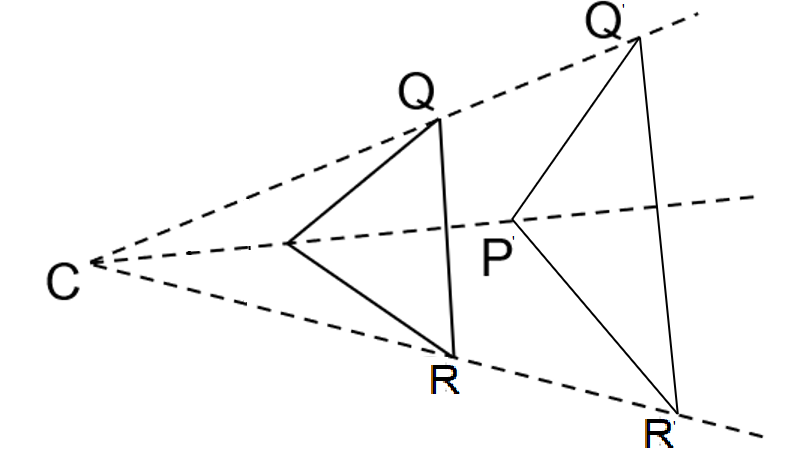
The above process was enlargement and now we do the reduction.
Step: 1 Now to get the triangle in between the point C and given
Step: 2 We will set our compass to a distance that is more than halfway between vertex P and points C. Step: 3 Keeping the compass at that same distance, we draw a curve that intersects the line between P and C. We do the same thing for all other points. Let’s assume the new vertices are
Step: 4 We connect the points
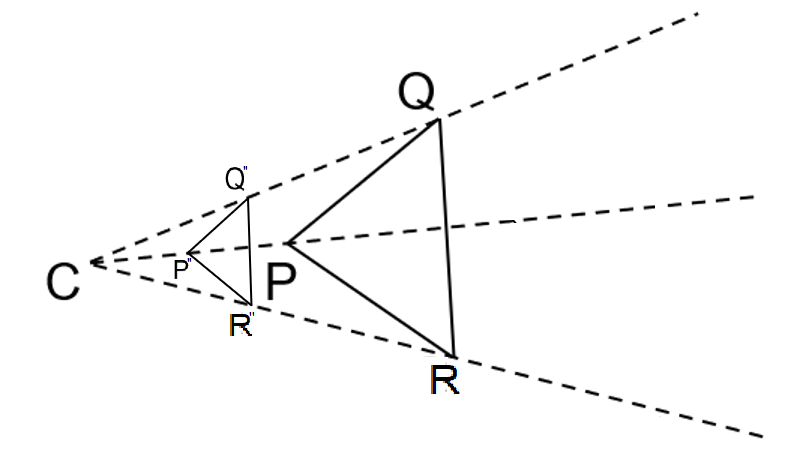
Note: We need to remember that one process is enough to show the dilation. This process is used to minimize and maximize the size of the triangle keeping all the respective side and angles ratios the same. These triangles are called similar triangles.
Complete step-by-step solution:
We follow some steps for the dilation process to dilate
Step: 1
We connect the center of dilation C to each vertex and extend those lines.
Step: 2
Then we place the point of the compass on the center of dilation C and the pencil on vertex Q to measure the distance.
Step: 3
Now, without changing the size of the compass, we will move the point of the compass to vertex Q, and make a mark on the line of extended CQ.
Step: 4
We now repeat the process for each of the other vertices of the triangle. Let’s assume the new vertices are
Step: 5
We connect the points

The above process was enlargement and now we do the reduction.
Step: 1 Now to get the triangle in between the point C and given
Step: 2 We will set our compass to a distance that is more than halfway between vertex P and points C. Step: 3 Keeping the compass at that same distance, we draw a curve that intersects the line between P and C. We do the same thing for all other points. Let’s assume the new vertices are
Step: 4 We connect the points

Note: We need to remember that one process is enough to show the dilation. This process is used to minimize and maximize the size of the triangle keeping all the respective side and angles ratios the same. These triangles are called similar triangles.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
EMI starts from ₹3,108.34 per month
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

What does R mean in math class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





