
Construction of a triangle of sides 4
Answer
529.2k+ views
Hint: Here we will proceed by practical geometry, using a set of rulers, compasses and protractors, different shapes. Then by applying the conditions given in the question we will get our answer.
Complete step-by-step answer:
Steps of construction are:
1. Firstly draw a line segment
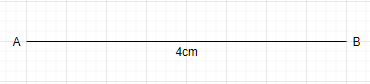
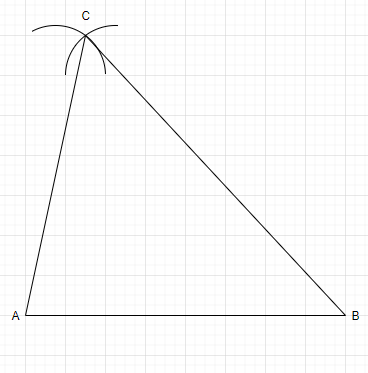
2. Now cut an arc of radius 5
3. Name the point of intersection of arcs to be point

4. Now join point
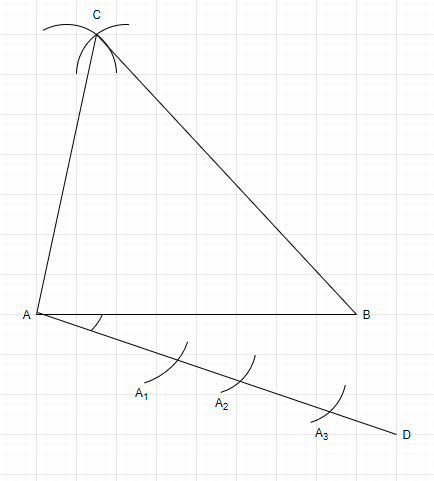
5. Draw a line

6. Cut three equal parts of line
7. Now join
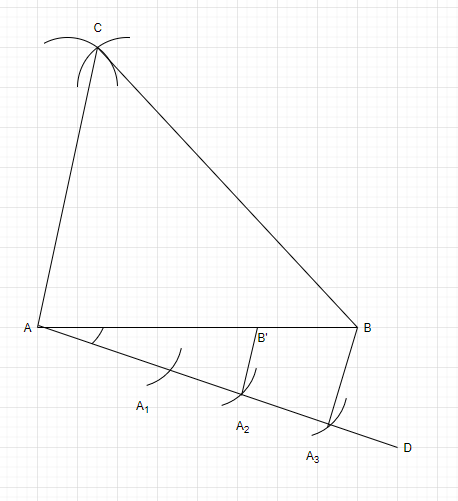
8. And then draw a line
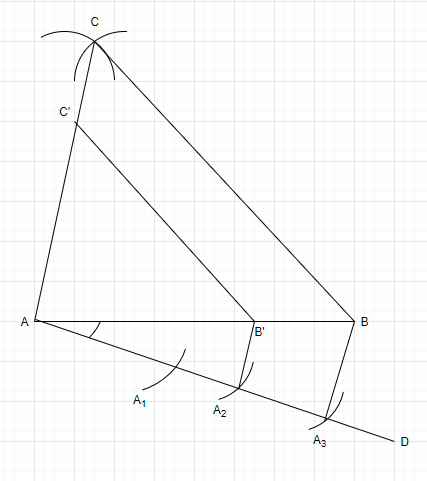
Hence,
Note: Whenever we come up with this type of problem, it is to be noted that in the construction of a triangle the three-sides and angle may or may not be equal in dimensions. Then by writing down the steps one by one we will get our required answer.
Complete step-by-step answer:
Steps of construction are:
1. Firstly draw a line segment

2. Now cut an arc of radius 5
3. Name the point of intersection of arcs to be point

4. Now join point

5. Draw a line

6. Cut three equal parts of line

7. Now join

8. And then draw a line

Hence,
Note: Whenever we come up with this type of problem, it is to be noted that in the construction of a triangle the three-sides and angle may or may not be equal in dimensions. Then by writing down the steps one by one we will get our required answer.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




