Question

Define the magnifying power of a microscope in terms of visual angle.

Answer 1

Hint: The process of magnification can occur in lenses, telescopes, microscopes, and even in slide projectors. Simple magnifying lenses are biconvex -these lenses are thicker in the middle than at the sides.

Complete step by step solution:
The magnifying glass was one of the first-ever instruments which were used to magnify an object. Magnification relates to the scaling up of images to see the fine details by increasing the image resolution. There are mainly two types of magnification - linear and angular:
Linear or transverse magnification – This happens for real images, like those projected on screens.
Angular magnification – This sort of magnification occurs in optical instruments that contain an eyepiece. The size of the image is determined by the angle subtended by the object at the focal point.
The magnifying power of an instrument is the ratio between the angular size of the image, as seen through the instrument, and the angular size of the object as seen by the naked eye.
Magnifying power $\left( m \right)$ a microscope is defined as the ratio of the visual angle subtended by the image at the eye $\left( \beta \right)$ to the visual angle subtended by the object at the eye when placed a minimum of the distance of distinct vision$\left( \alpha \right).$
We can refer to following the figure for further understanding.

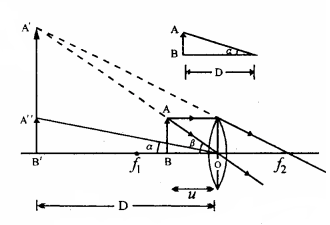
$\therefore {\text{m = }}\dfrac{\beta }{\alpha }$
Where $\beta $ is the visual angle of the image and $\alpha $ is the visual angle of the object, placed at the least distance of distinct vision.
As the angle $\alpha $ and$\beta $ are small therefore,
$\beta \approx \tan \beta $ and$\alpha \approx \tan \alpha $
$\therefore {\text{m = }}\dfrac{{\tan \alpha }}{{\tan \beta }}$
In $\Delta $ AOB, $\tan \beta = \dfrac{{{\text{AB}}}}{{{\text{OB}}}}$
And $\tan \alpha = \dfrac{{{\text{A''B'}}}}{{{\text{OB'}}}} = \dfrac{{{\text{AB}}}}{{{\text{OB'}}}}$ distinct vision.
$\Rightarrow m=\dfrac{tan \alpha}{tan \beta}$
In $\Delta AOB, tan \beta = \dfrac{AB}{OB}$
And, $tan \alpha=\dfrac{A’’B’}{OB’}=\dfrac{AB}{OB’}$ [Distinct vision]
$\Rightarrow =\dfrac{AB/OB}{AB/OB’}$
$\Rightarrow m=\dfrac{AB}{OB} \times \dfrac{OB’}{AB}=\dfrac{OB’}{AB}=\dfrac{D}{u}$
Now by using lens formula,
$\dfrac{1}{{\text{v}}} - \dfrac{1}{{\text{u}}} = \dfrac{1}{{\text{f}}}$
$ \Rightarrow \dfrac{1}{{{\text{( - D)}}}} - \dfrac{1}{{{\text{( - u)}}}} = \dfrac{1}{{\text{f}}}$
$ \Rightarrow \dfrac{1}{{\text{u}}} = \dfrac{{{\text{D + f}}}}{{{\text{Df}}}}$
$ \Rightarrow {\text{m = D}} \times \left( {\dfrac{{{\text{D + f}}}}{{{\text{Df}}}}} \right) = \left( {1 + \dfrac{{\text{D}}}{{\text{f}}}} \right)$

Note:
The mathematical formula for Magnifying Power, $M$ is
$M = \dfrac{D}{f}$ when the image forms at near point.
And also,
$M = 1 + \dfrac{D}{f}$ when the image forms at infinity.
Where $D$ is the least distance of distinct vision and is equal to $v$. $D$ being constant and the magnifying power depends upon the focal length of the lens. Smaller the focal length is going greater will be the magnifying power of the lens.