
Derive Bragg’s equation.
Answer
430.1k+ views
Hint: Bragg’s Law explains the molecular structure and crystals being identified using X-ray diffraction techniques. It gives us the relationship between the incident X-ray light and its reflection from the crystal surface.
Complete step by step answer:
-Bragg’s Law was first given by Sir W.H. Bragg and his son Sir W.L. Bragg.
-This law simply states that when the X-ray is incident onto a crystal surface, its angle of incidence is ‘
-Bragg’s equation is:
-To derive this equation, first understand the following diagram:
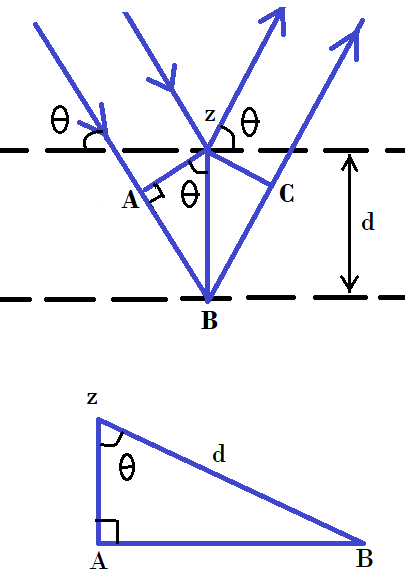
When the incident angle equals the reflecting angle, the phases of the 2 beams of X-ray coincide. The 2 beams of incident ray are parallel till point z. Point z is the point where the top beam strikes the top layer. The next beam passes up to the next layer and is scattered by B. This beam has travelled an extra distance of AB+BC than the previous one. This distance is an integral multiple of the wavelength of the ray.
So,
Hence we can say:
In triangle ABz: d is the hypotenuse and AB is opposite to angle
Where P=perpendicular and H=hypotenuse
Here,
Hence,
Now put the value of AB obtained in equation (2) in equation (1). We get:
This is Bragg's equation. Hence derived.
Note: Remember that Bragg’s law is valid only if both the angle of incidence and the angle of reflection are equal to each other and the path difference is equal to an integer number of wavelengths. If these conditions are satisfied constructive interference will occur.
Complete step by step answer:
-Bragg’s Law was first given by Sir W.H. Bragg and his son Sir W.L. Bragg.
-This law simply states that when the X-ray is incident onto a crystal surface, its angle of incidence is ‘
-Bragg’s equation is:
-To derive this equation, first understand the following diagram:

When the incident angle equals the reflecting angle, the phases of the 2 beams of X-ray coincide. The 2 beams of incident ray are parallel till point z. Point z is the point where the top beam strikes the top layer. The next beam passes up to the next layer and is scattered by B. This beam has travelled an extra distance of AB+BC than the previous one. This distance is an integral multiple of the wavelength of the ray.
So,
Hence we can say:
In triangle ABz: d is the hypotenuse and AB is opposite to angle
Where P=perpendicular and H=hypotenuse
Here,
Hence,
Now put the value of AB obtained in equation (2) in equation (1). We get:
This is Bragg's equation. Hence derived.
Note: Remember that Bragg’s law is valid only if both the angle of incidence and the angle of reflection are equal to each other and the path difference is equal to an integer number of wavelengths. If these conditions are satisfied constructive interference will occur.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Draw a diagram of a flower and name the parts class 12 biology ICSE

The speed of light will be minimum while passing through class 12 physics CBSE

For the angle of minimum deviation of a prism to be class 12 physics CBSE

If Meselson and Stahls experiment is continued for class 12 biology CBSE

Identify the functional group in the given compoun class 12 chemistry CBSE

Two reactions A to products and B to products have class 12 chemistry CBSE




