
How do you determine if
Answer
390.9k+ views
Hint: In trigonometry
Complete step by step answer:
In basic trigonometry,
The relations of these line segments of the triangle are expressed with three specific functions known as Sine, Cosine and Tangent.
Now, we consider a right triangle, the angle made by the base and hypotenuse will be considered as
Therefore,
Here, in this question we have to determine whether
So, let us consider a right- triangle angle where the angle made by the base and hypotenuse
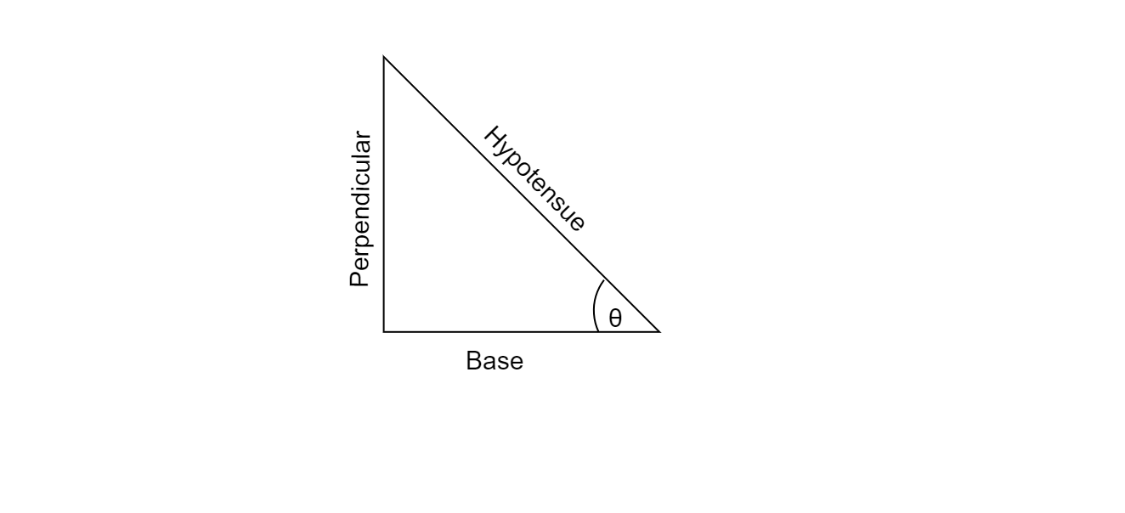
Now, if we increase the angle
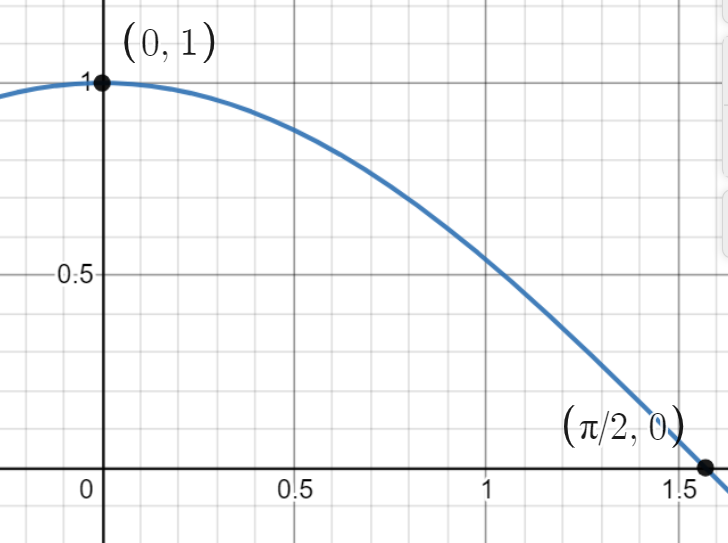
Therefore, cosine is a decreasing function and it is also clear from the values of cosine angles i.e.,
Hence, the given statement is False.
The correct statement will be
Note:
Trigonometric functions also known as circular functions are the functions or ratios which state the relationship between the angles and side of the right- angled triangle. The basic trigonometric functions are sine, cosine, tangent, secant, cosecant and cotangent. In these trigonometric functions
Complete step by step answer:
In basic trigonometry,
The relations of these line segments of the triangle are expressed with three specific functions known as Sine, Cosine and Tangent.
Now, we consider a right triangle, the angle made by the base and hypotenuse will be considered as
Therefore,
Here, in this question we have to determine whether
So, let us consider a right- triangle angle where the angle made by the base and hypotenuse

Now, if we increase the angle

Therefore, cosine is a decreasing function and it is also clear from the values of cosine angles i.e.,
Hence, the given statement is False.
The correct statement will be
Note:
Trigonometric functions also known as circular functions are the functions or ratios which state the relationship between the angles and side of the right- angled triangle. The basic trigonometric functions are sine, cosine, tangent, secant, cosecant and cotangent. In these trigonometric functions
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE




