
How do you determine whether each equation is a linear equation:
Answer
455.7k+ views
Hint: In this question, we have to determine whether a given equation is a linear equation. For this, we have to make a given equation in the form of slope intercept form of a line. It can be done by first dividing both sides of the equation by
The Slope Intercept Form of a Line:
The equation of a line with slope
Complete step by step solution:
We know that the slope intercept form of a line is the equation of a line with slope
Given equation is
So, we have to make a given equation in the form of
First, divide both sides of the equation by
Since, our equation has exponents, it is a nonlinear equation.
Hence,
Note: We can also check whether a given equation is a linear equation by plotting the equation.
Then, determine whether the graph is straight line or curved.
If the line is straight, the equation is linear. If it is curved, it is a nonlinear equation.
Graph of
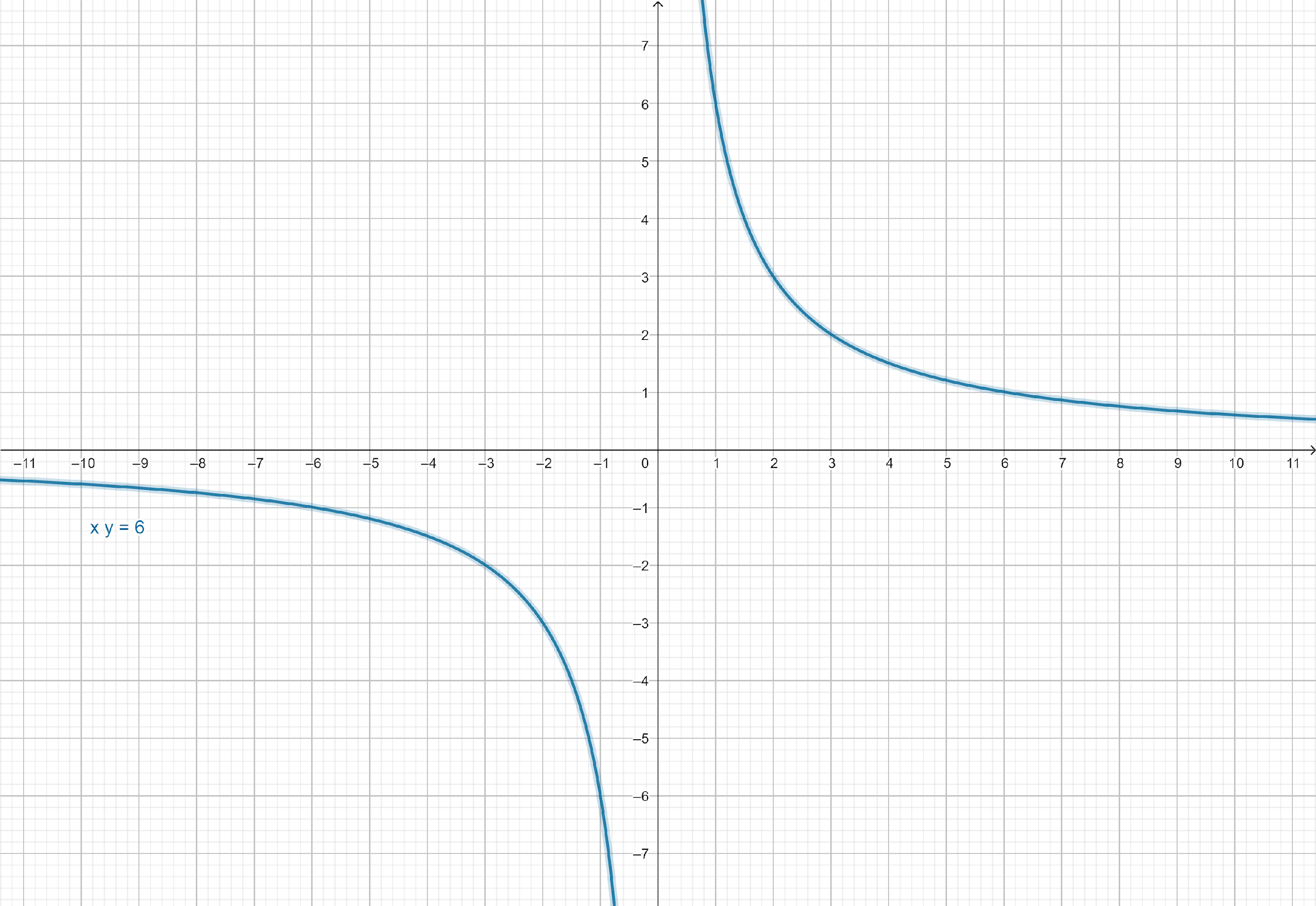
From the graph, we can observe that
The Slope Intercept Form of a Line:
The equation of a line with slope
Complete step by step solution:
We know that the slope intercept form of a line is the equation of a line with slope
Given equation is
So, we have to make a given equation in the form of
First, divide both sides of the equation by
Since, our equation has exponents, it is a nonlinear equation.
Hence,
Note: We can also check whether a given equation is a linear equation by plotting the equation.
Then, determine whether the graph is straight line or curved.
If the line is straight, the equation is linear. If it is curved, it is a nonlinear equation.
Graph of

From the graph, we can observe that
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




