
Draw a circle of radius 5cm. From a point 13cm away from the centre, construct a pair of tangents to the circle and measure their length. Also verify the measurement by actual calculation.
Answer
443.1k+ views
Hint: We need to construct a pair of tangents to a circle and find their length. We start to solve the given question by constructing a circle of radius 5cm with centre O and draw a line segment OP of length 13cm. Then, we need to find the length of the tangent of the circle. Lastly, we verify the length of the tangent to a circle by actual calculation.
Complete step by step solution:
We are asked to draw a circle of radius 5 cm and need to construct tangents for the same. We will be solving the given question by constructing a circle of radius 5 cm and then finding the length of tangents to the circle.
The tangent to the circle is defined as the straight line that touches a circle at exactly one point. The point of contact where a tangent meets the circle is called tangency or point of contact. There can be only one tangent to a circle at a given point.
The pair of tangents to a circle can be constructed as follows,
1. We need to draw a circle of radius 5cm with centre O using a compass and pencil. So, we can take 5 cm on the compass and draw the circle as below,
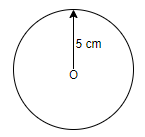
2.Draw a line segment OP of length 13 cm from the centre of the circle to P.
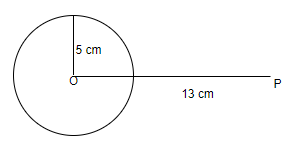
3. Join point P to any point Q on the circle such that PQ touches the circle.
After the steps, we find that the length of PQ is 12 cm with the help of a ruler.
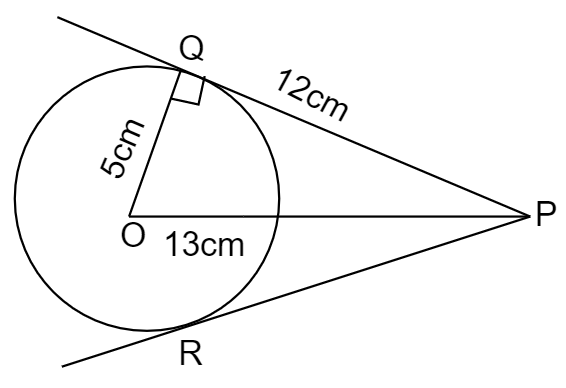
Actual calculation:
The above steps can be diagrammatically represented as follows,
In the above figure,
PQ and PR are the pair of tangents to a circle.
We need to find the length of tangents PQ, PR.
From the figure,
We know that a triangle
Applying the Pythagoras theorem to the triangle, we get,
Here,
Substituting the same, we get,
Simplifying the above equation, we get,
The length of a tangent cannot be negative.
Note: We need to precisely measure the length of the sides with the ruler. We must remember that the value of
Complete step by step solution:
We are asked to draw a circle of radius 5 cm and need to construct tangents for the same. We will be solving the given question by constructing a circle of radius 5 cm and then finding the length of tangents to the circle.
The tangent to the circle is defined as the straight line that touches a circle at exactly one point. The point of contact where a tangent meets the circle is called tangency or point of contact. There can be only one tangent to a circle at a given point.
The pair of tangents to a circle can be constructed as follows,
1. We need to draw a circle of radius 5cm with centre O using a compass and pencil. So, we can take 5 cm on the compass and draw the circle as below,

2.Draw a line segment OP of length 13 cm from the centre of the circle to P.

3. Join point P to any point Q on the circle such that PQ touches the circle.
After the steps, we find that the length of PQ is 12 cm with the help of a ruler.

Actual calculation:
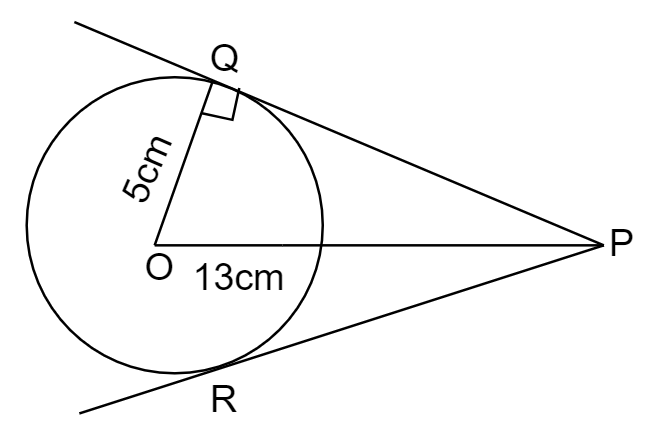
The above steps can be diagrammatically represented as follows,

In the above figure,
PQ and PR are the pair of tangents to a circle.
We need to find the length of tangents PQ, PR.
From the figure,
We know that a triangle
Applying the Pythagoras theorem to the triangle, we get,
Here,
Substituting the same, we get,
Simplifying the above equation, we get,
The length of a tangent cannot be negative.
Note: We need to precisely measure the length of the sides with the ruler. We must remember that the value of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




