Question

Draw a circuit diagram to verify ohm’s law.

Answer 1

Hint: In this question, we need to verify the ohm's law by sketching a circuit diagram. For this, we will follow Kirchhoff's voltage law.

Complete step by step answer:Let us consider a circuit in which a resistance “R” is connected with a DC voltage source, “V”.

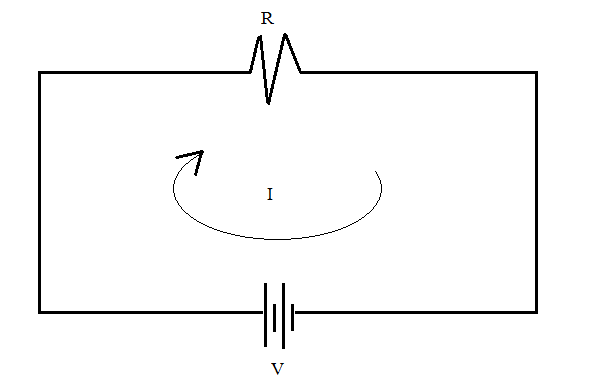
As the circuit is closed, a current of magnitude “I” flows in the circuit.
According to Kirchhoff’s voltage law, the sum of the voltage drop across all the elements attached in the closed-circuit is arithmetical equals to zero.
So, in the above figure, there is a voltage source of magnitude “V”. There will be the voltage drop which will be equivalent to the product of the current flowing through the resistance and the magnitude of the resistance. Mathematically, ${V_r} = IR$ where “I” is the current flowing through the resistance “R” and ${V_r}$ is the potential drop across the resistance.
Now, applying Kirchhoff’s voltage law, we get
$
  V - IR = 0 \\
  V = IR \\
 $
The above equation can also be written as:
$\dfrac{V}{I} = R$
Here, “R” is the constant value which is fixed and will not change with time and the voltage source connected across it. Hence, we can write $\dfrac{V}{I} = R$ as:
$\dfrac{V}{I} = {\text{constant}}$
The above equation can again be re-written as:
$V \propto I$
This is the basic statement and the mathematical expression for the ohm’s law which states that the voltage in a closed circuit is directly proportional to the current associated with the circuit only.

Note:Students must know about the Kirchhoff’s voltage law to establish the ohm’s law. Moreover, it is interesting to note here that the ohm’s law is not valid for the semiconductor devices.