
Draw the angle using ruler and compasses. Also, label them.
${{45}^{\circ }}$
Answer
492.9k+ views
Hint: In order to solve this problem, we have to use a compass and ruler. We need to draw an angle of ${{45}^{\circ }}$ . We need to first draw an angle of ${{90}^{\circ }}$ first then followed by bisecting it. It is not possible to draw an angle of ${{45}^{\circ }}$ only with the help of a compass. Not all angles can be drawn without the help of a protractor.
Complete step-by-step answer:
We need to draw an acute angle of ${{45}^{\circ }}$with the help of a ruler and compass.
An angle is called acute when the angle made is always less than ${{90}^{\circ }}$ .
Let us consider we need to draw an angle of ${{45}^{\circ }}$ namely $\angle ABC$ .
For that, we need to start by drawing a horizontal segment of BC

Also, we need to keep some space upwards so that our angle fits in that space.
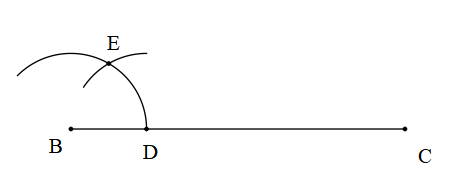
Taking some approximate distance in the compass draws an arc greater than a quarter circle.
The point intersecting line BC names it as D.
It is shown in the figure as below,

Keeping the same distance in compass, from point D draw an arc on the previous arc.
Name the point of intersection as E.
It is shown as below,
Keeping the same distance in compass, from point E draw an arc on the original arc.
Name the point of intersection as F.
It is shown as below,
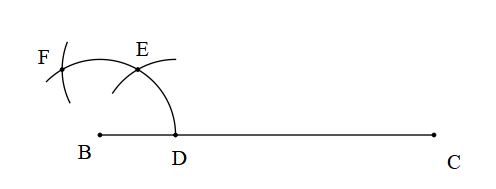
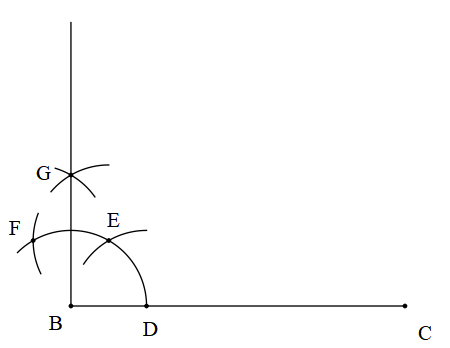
Now with the same distance, keeping compass on E, draw in the region of E and F towards the upper side of segment BC.
And repeat the process from point F.
Make sure that both the new arcs intersect each other and name the point of intersection as G.
It is shown as below,

Connect the points B and G by a segment.
The angle between BG and BC is ${{90}^{\circ }}$ .
It is shown as below,
Now as we know that half of ${{90}^{\circ }}$ is ${{45}^{\circ }}$ .
Therefore, we need to bisect this angle.
Name the intersection of BG with arc DF as point H.
Keeping compass on point D and taking distance more than half of BC, draw an arc in the middle of this right angle.
Repeat the same process by keeping the compass at point H without changing the distance in the compass.
Name the point of intersection as point A.
It is shown as below,
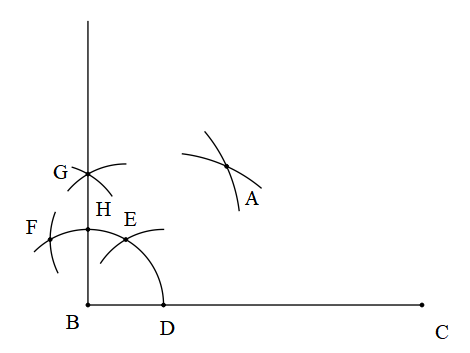
By connecting the points A and B by a line segment, we get $\angle ABC$ as ${{45}^{\circ }}$ .
The final diagram after construction is as follows,
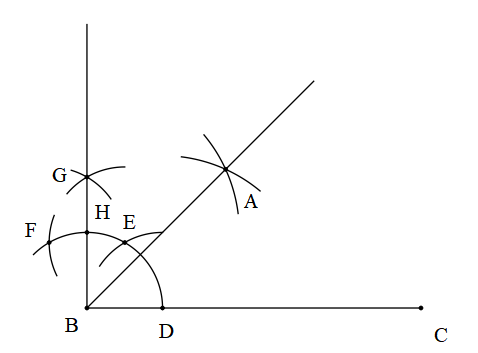
Hence, this is the required solution.
Note: It is always important to name all the points of intersection. Also While drawing the angle of ${{90}^{\circ }}$, we must not change the distance in the compass. We can check the angle with the help of a protractor. Segment AB is also called the angle bisector of $\angle GBC$ .
Complete step-by-step answer:
We need to draw an acute angle of ${{45}^{\circ }}$with the help of a ruler and compass.
An angle is called acute when the angle made is always less than ${{90}^{\circ }}$ .
Let us consider we need to draw an angle of ${{45}^{\circ }}$ namely $\angle ABC$ .
For that, we need to start by drawing a horizontal segment of BC

Also, we need to keep some space upwards so that our angle fits in that space.
Taking some approximate distance in the compass draws an arc greater than a quarter circle.
The point intersecting line BC names it as D.
It is shown in the figure as below,

Keeping the same distance in compass, from point D draw an arc on the previous arc.
Name the point of intersection as E.
It is shown as below,

Keeping the same distance in compass, from point E draw an arc on the original arc.
Name the point of intersection as F.
It is shown as below,
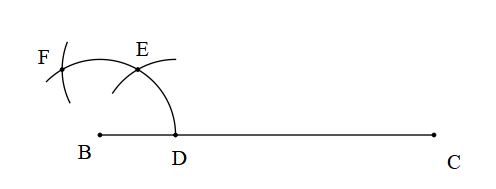
Now with the same distance, keeping compass on E, draw in the region of E and F towards the upper side of segment BC.
And repeat the process from point F.
Make sure that both the new arcs intersect each other and name the point of intersection as G.
It is shown as below,

Connect the points B and G by a segment.
The angle between BG and BC is ${{90}^{\circ }}$ .
It is shown as below,

Now as we know that half of ${{90}^{\circ }}$ is ${{45}^{\circ }}$ .
Therefore, we need to bisect this angle.
Name the intersection of BG with arc DF as point H.
Keeping compass on point D and taking distance more than half of BC, draw an arc in the middle of this right angle.
Repeat the same process by keeping the compass at point H without changing the distance in the compass.
Name the point of intersection as point A.
It is shown as below,

By connecting the points A and B by a line segment, we get $\angle ABC$ as ${{45}^{\circ }}$ .
The final diagram after construction is as follows,

Hence, this is the required solution.
Note: It is always important to name all the points of intersection. Also While drawing the angle of ${{90}^{\circ }}$, we must not change the distance in the compass. We can check the angle with the help of a protractor. Segment AB is also called the angle bisector of $\angle GBC$ .
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Choose the correct assertive sentence form of the below class 7 english CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

What are the controls affecting the climate of Ind class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Write a letter to the editor of the national daily class 7 english CBSE





