
Estimate the area of the irregular shape. Explain your method and show your work?
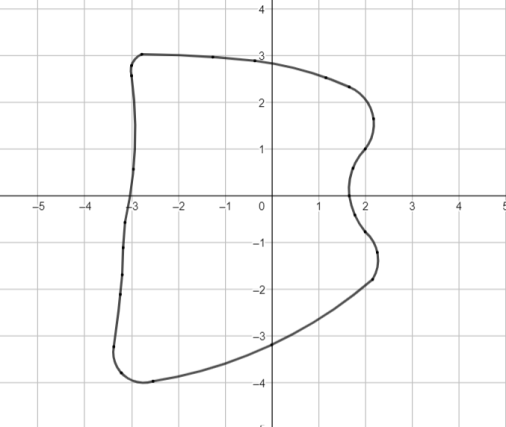

Answer
448.5k+ views
Hint: As the figure is a little difficult to figure out so, we will line them up with straight lines. After this we will apply the formulas
Complete step by step solution:
If we look at the given diagram carefully, then we come to know that it looks similar to the shape of a trapezium. To clear this point, we will understand about some properties of trapezium. Every trapezium will have one thing common which is its parallel side. This means that any trapezium will have one side parallel to the other side. This is the only pair of parallel sides a trapezium will have.
If we look at the diagram carefully, then it is difficult to imagine its shape. But if we somehow create straight lines approximately, then we can assume it as a trapezium. This can be understood from the following figure.
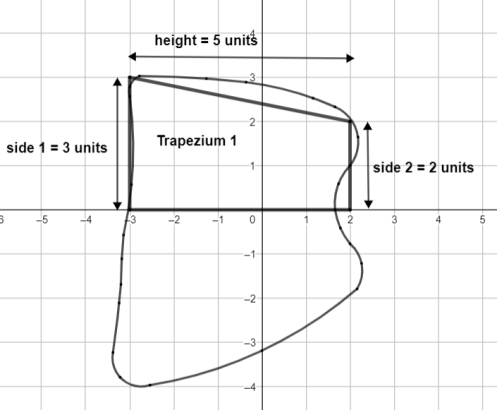
So, we can find the area of trapezium 1. For this we will take the help of the formula of trapezium,
Similarly, we can apply this formula to the second trapezium as below.
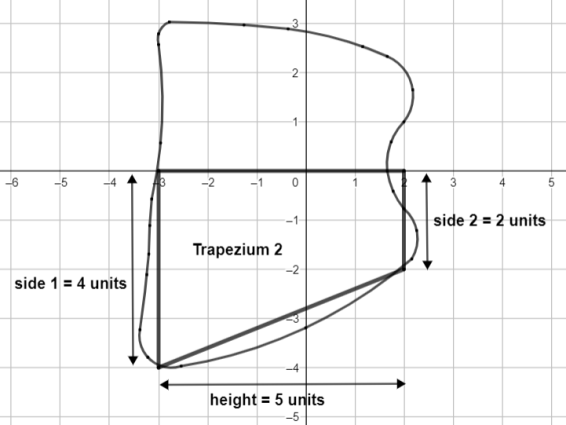
So, we can find the area of trapezium 2. For this we will take the help of the formula of trapezium,
We can see in this above figure that there is some region left on the left side of the figure. This must be covered too. After approximately lining up this space, we come to know that it is a triangle as shown below.
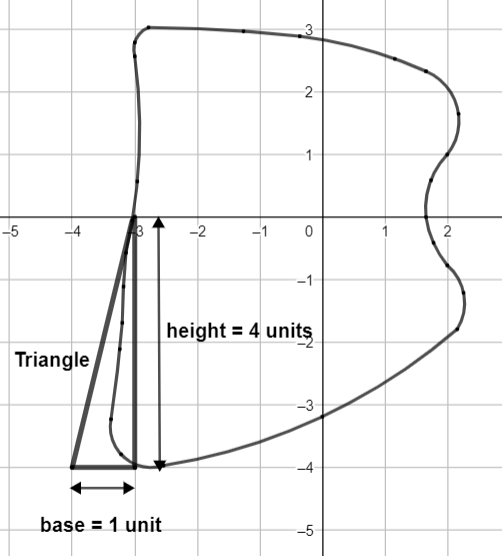
To find the area of the triangle as marked in the above diagram, we will apply the formula of it as
Now, we will add up all the areas as in, equation (ii) + equation (iii) + equation (iv). Thus, we get
Hence, the total area of the given region is 29.5 units.
Note: Sometimes, it becomes difficult for us to figure out the shape of such diagrams which are not even defined to us. For example in this diagram we have a drawing which cannot be figured out at first. So, we will apply approximately straight lines and get to the result. Here we come to know that the diagram that is made after using straight lines is trapezium and a little triangle. After this we will use the formulas of trapezium and triangle. To get the area of the whole figure we need to add up all the small areas and get to the desired result. One should focus on the question while solving otherwise, there might be a chance to get a wrong solution.
Complete step by step solution:
If we look at the given diagram carefully, then we come to know that it looks similar to the shape of a trapezium. To clear this point, we will understand about some properties of trapezium. Every trapezium will have one thing common which is its parallel side. This means that any trapezium will have one side parallel to the other side. This is the only pair of parallel sides a trapezium will have.
If we look at the diagram carefully, then it is difficult to imagine its shape. But if we somehow create straight lines approximately, then we can assume it as a trapezium. This can be understood from the following figure.

So, we can find the area of trapezium 1. For this we will take the help of the formula of trapezium,
Similarly, we can apply this formula to the second trapezium as below.

So, we can find the area of trapezium 2. For this we will take the help of the formula of trapezium,
We can see in this above figure that there is some region left on the left side of the figure. This must be covered too. After approximately lining up this space, we come to know that it is a triangle as shown below.

To find the area of the triangle as marked in the above diagram, we will apply the formula of it as
Now, we will add up all the areas as in, equation (ii) + equation (iii) + equation (iv). Thus, we get
Hence, the total area of the given region is 29.5 units.
Note: Sometimes, it becomes difficult for us to figure out the shape of such diagrams which are not even defined to us. For example in this diagram we have a drawing which cannot be figured out at first. So, we will apply approximately straight lines and get to the result. Here we come to know that the diagram that is made after using straight lines is trapezium and a little triangle. After this we will use the formulas of trapezium and triangle. To get the area of the whole figure we need to add up all the small areas and get to the desired result. One should focus on the question while solving otherwise, there might be a chance to get a wrong solution.
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




