Question

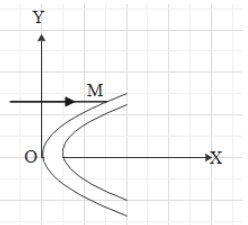
Figure shows a parabolic mirror with the equation of section on the XY plane as \[{{y}^{2}}=4ax\]. A light ray parallel to X-axis is incident on the mirror at a point M with abscissa 4a. The unit vector along the reflected ray will be
                       

\[\begin{align}
  & \text{A) }\dfrac{1}{5}\left( 4\overset{\wedge }{\mathop{i}}\,+3\overset{\wedge }{\mathop{j}}\, \right) \\
 & \text{B) }\dfrac{1}{5}\left( 4\overset{\wedge }{\mathop{i}}\,-3\overset{\wedge }{\mathop{j}}\, \right) \\
 & \text{C) }\dfrac{1}{5}\left( 3\overset{\wedge }{\mathop{i}}\,+4\overset{\wedge }{\mathop{j}}\, \right) \\
 & \text{D) }\dfrac{1}{5}\left( 3\overset{\wedge }{\mathop{i}}\,-4\overset{\wedge }{\mathop{j}}\, \right) \\
\end{align}\]

Answer 1

Hint: Here we have to find the unit vector along the reflected ray. We have given abscissa which is the coordinate of X-axis at the point of intersection i.e. point at which light is incident. An equation of parabola for the given case. So by using the parabolic equation we can find the Y-coordinate. Once we find the coordinate and apply the laws of reflection for spherical mirrors and by finding slope we can find the unit vector.

Formula used:
\[\overrightarrow{u}=\dfrac{a\overset{\wedge }{\mathop{i}}\,+b\overset{\wedge }{\mathop{j}}\,}{\sqrt{{{a}^{2}}+{{b}^{2}}}}\]

Complete answer:
Parabolic mirror can be convex mirror or concave mirror, here in the given question we have convex mirror. And here X-axis is the principal axis and Y-axis is the pole of the mirror. Now we know that the ray travelling parallel to the axis gets reflected and passes from the focus. But here the given mirror is a convex mirror and it will diverge after reflecting and the extended line will pass from the focus.
Now for the parabola having equation \[{{y}^{2}}=4ax\], the focus is given by the coordinates (a,0). So for the given parabolic mirror focus will be at (a,0) and the extended line for the reflected ray will pass from the (a,0). The given diagram can we redrawn as

MN is the reflected ray and F is the focus. M is the point of intersection where the incident ray and parabolic mirror intersect and its abscissa was given 4a (abscissa is the X coordinate for the point M). We can find the Y-coordinate for point M by substituting the value of x in the parabolic equation\[{{y}^{2}}=4ax\]. (Value of x is the 4a)
Hence we can write
\[\begin{align}
  & {{y}^{2}}=4ax \\
 & \Rightarrow {{y}^{2}}=4a(4a) \\
 & \Rightarrow {{y}^{2}}=16{{a}^{2}} \\
 & \Rightarrow y=\sqrt{16{{a}^{2}}} \\
 & \Rightarrow y=4a \\
\end{align}\]
Hence the coordinates for point M is (4a, 4a) which is already marked in the above diagram.
Now we have to find the unit vector for the reflected ray and the unit vector for equation of line \[ax-by+c=0\] will be
\[\overrightarrow{u}=\dfrac{a\overset{\wedge }{\mathop{i}}\,+b\overset{\wedge }{\mathop{j}}\,}{\sqrt{{{a}^{2}}+{{b}^{2}}}}\]
Here a is the rise along X-axis and b is rise along Y-axis and therefore the slope for the equation of line is given as
\[m=\dfrac{a}{b}\text{ }................\text{(i)}\]
Now FM and MN are the same line so it will have the same equation of line and same slope. If \[({{x}_{F}},{{y}_{F}})\]are coordinates at point F and \[({{x}_{M}},{{y}_{M}})\]are coordinates at point M, then slope for FM can be given as
\[\begin{align}
  & m=\dfrac{{{y}_{F}}-{{y}_{M}}}{{{x}_{F}}-{{x}_{M}}} \\
 & m=\dfrac{4a-0}{4a-a} \\
 & m=\dfrac{4a}{3a} \\
 & m=\dfrac{4}{3} \\
\end{align}\]
Comparing the above equation with equation (i), \[a=4\text{ and }b=3\]
So the unit vector for reflected ray can we given as
\[\begin{align}
  & \overrightarrow{u}=\dfrac{a\overset{\wedge }{\mathop{i}}\,+b\overset{\wedge }{\mathop{j}}\,}{\sqrt{{{a}^{2}}+{{b}^{2}}}} \\
 & \Rightarrow \overrightarrow{u}=\dfrac{4\overset{\wedge }{\mathop{i}}\,+3\overset{\wedge }{\mathop{j}}\,}{\sqrt{{{4}^{2}}+{{3}^{2}}}} \\
 & \Rightarrow \overrightarrow{u}=\dfrac{4\overset{\wedge }{\mathop{i}}\,+3\overset{\wedge }{\mathop{j}}\,}{\sqrt{16+9}} \\
 & \Rightarrow \overrightarrow{u}=\dfrac{4\overset{\wedge }{\mathop{i}}\,+3\overset{\wedge }{\mathop{j}}\,}{\sqrt{25}} \\
 & \Rightarrow \overrightarrow{u}=\dfrac{4\overset{\wedge }{\mathop{i}}\,+3\overset{\wedge }{\mathop{j}}\,}{5} \\
 & \Rightarrow \overrightarrow{u}=\dfrac{1}{5}\left( 4\overset{\wedge }{\mathop{i}}\,+3\overset{\wedge }{\mathop{j}}\, \right) \\
\end{align}\]

Hence option A is the correct option.

Note:
FM and MN represents the same line as FM is the extended line of the reflected ray MN. In case of concave mirror light ray will be incident of the inner surface but it will pass through the same focus after reflection so the unit vector for reflected ray will be the same.
Like X-coordinate is called abscissa same way, Y-coordinate is also called as ordinate.