
Figure shows a semicircle that is the graph of the equation
A.
B.
C.
D.
E.
Answer
475.8k+ views
Hint: We denote the other point of intersection except origin as A. We find the coordinate of A by finding the solutions of
Complete step-by-step solution:
We see in the figure that a semicircle is present whose one end is at the origin is defined by the equation
We denote the other end of the semicircle lying on the positive
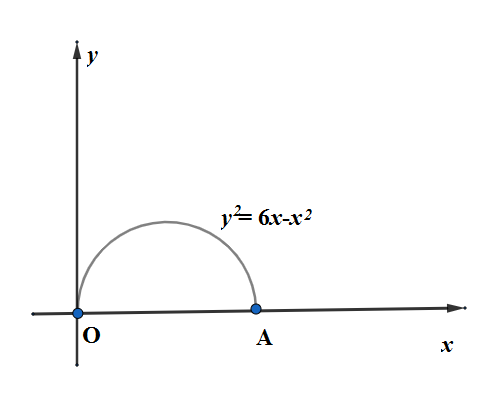
Since the equation of
We square both sides of above equation to have;
We factorize by taking
Since the product of the two factors is zero one of them must be zero. So we have;
So we have two roots of the equation
We are given the question that the semicircle is rotated
So the correct option is E.
Note: We note that the question presumes that the semicircle is not displaced from the original end points O and A when it's rotated about the
Complete step-by-step solution:
We see in the figure that a semicircle is present whose one end is at the origin is defined by the equation
We denote the other end of the semicircle lying on the positive
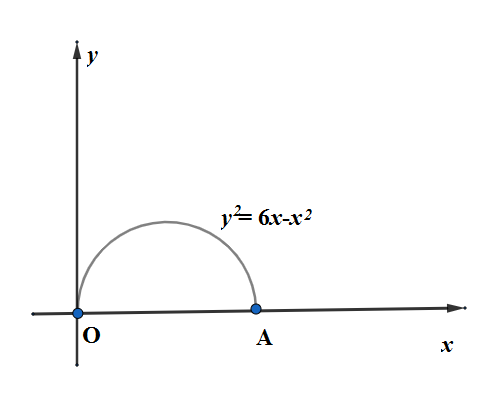
Since the equation of
We square both sides of above equation to have;
We factorize by taking
Since the product of the two factors is zero one of them must be zero. So we have;
So we have two roots of the equation
We are given the question that the semicircle is rotated
So the correct option is E.
Note: We note that the question presumes that the semicircle is not displaced from the original end points O and A when it's rotated about the
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE

SI unit of electrical energy is A Joule B Kilowatt class 10 physics CBSE




