
Find the angle measure of

Answer
475.5k+ views
1 likes
Hint: Here, we need to find the value of
Complete step-by-step answer:
(a)
The sum of all the interior angles of a quadrilateral is equal to
Therefore, we get
This is a linear equation in terms of
Adding the terms of the equation, we get
Subtracting
Thus, we get
Therefore, we get the value of
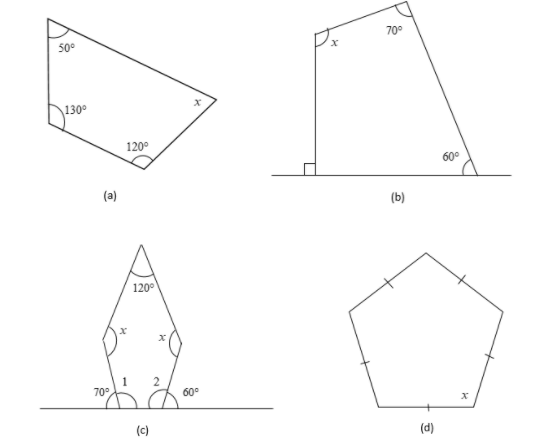
(b)
First, we will mark another angle in the figure.
The sum of all the angles lying on a line is equal to
From the figure, we can observe that the right angle and angle 1 form a linear pair.
Therefore, we get
Subtracting
The sum of all the interior angles of a quadrilateral is equal to
Therefore, we get
This is a linear equation in terms of
Substituting
Adding the terms of the equation, we get
Subtracting
Thus, we get
Therefore, we get the value of
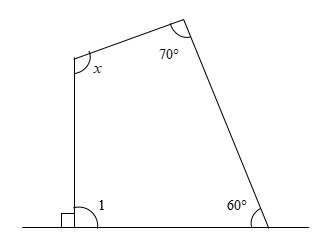
(c)
First, we will mark two angles in the figure.
The sum of all the angles lying on a line is equal to
From the figure, we can observe that the angle measuring
Therefore, we get
Subtracting
From the figure, we can observe that the angle measuring
Therefore, we get
Subtracting
The sum of all the interior angles of a pentagon is equal to
Therefore, we get
This is a linear equation in terms of
Substituting
Adding the terms of the equation, we get
Subtracting
Dividing both sides of the equation by 2, we get
Therefore, we get the value of
(d)
It is shown that all sides of the pentagon are equal.
Therefore, the given pentagon is a regular pentagon.
We know that all the sides and interior angles of a regular polygon are equal.
Therefore, we get the measure of the five interior angles as
The sum of all the interior angles of a pentagon is equal to
Therefore, we get
This is a linear equation in terms of
Adding the terms of the equation, we get
Dividing both sides of the equation by 5, we get
Therefore, we get the value of
Note: We have formed linear equations in one variable in terms of
We used the term ‘regular polygon’ in the solution. A polygon is a closed figure made using straight lines as sides. A regular polygon is a polygon whose interior angles and sides are equal. For example: a square is a regular polygon having 4 sides, a pentagon is a regular polygon having 5 sides, a hexagon is a regular polygon having 6 sides, etc.
Complete step-by-step answer:
(a)
The sum of all the interior angles of a quadrilateral is equal to
Therefore, we get
This is a linear equation in terms of
Adding the terms of the equation, we get
Subtracting
Thus, we get
Therefore, we get the value of
(b)
First, we will mark another angle in the figure.

The sum of all the angles lying on a line is equal to
From the figure, we can observe that the right angle and angle 1 form a linear pair.
Therefore, we get
Subtracting
The sum of all the interior angles of a quadrilateral is equal to
Therefore, we get
This is a linear equation in terms of
Substituting
Adding the terms of the equation, we get
Subtracting
Thus, we get
Therefore, we get the value of
(c)
First, we will mark two angles in the figure.

The sum of all the angles lying on a line is equal to
From the figure, we can observe that the angle measuring
Therefore, we get
Subtracting
From the figure, we can observe that the angle measuring
Therefore, we get
Subtracting
The sum of all the interior angles of a pentagon is equal to
Therefore, we get
This is a linear equation in terms of
Substituting
Adding the terms of the equation, we get
Subtracting
Dividing both sides of the equation by 2, we get
Therefore, we get the value of
(d)
It is shown that all sides of the pentagon are equal.
Therefore, the given pentagon is a regular pentagon.
We know that all the sides and interior angles of a regular polygon are equal.
Therefore, we get the measure of the five interior angles as
The sum of all the interior angles of a pentagon is equal to
Therefore, we get
This is a linear equation in terms of
Adding the terms of the equation, we get
Dividing both sides of the equation by 5, we get
Therefore, we get the value of
Note: We have formed linear equations in one variable in terms of
We used the term ‘regular polygon’ in the solution. A polygon is a closed figure made using straight lines as sides. A regular polygon is a polygon whose interior angles and sides are equal. For example: a square is a regular polygon having 4 sides, a pentagon is a regular polygon having 5 sides, a hexagon is a regular polygon having 6 sides, etc.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




