
How do you find the area of the cross-section of a sphere formed by a plane intersecting the sphere at an equator, if the radius of the sphere is 11 inches?
Answer
465.9k+ views
Hint: Here the cross-section of the sphere which is formed by a plane that intersects the sphere at an equator will be a circle of the same radius as that of the sphere. So to find the area of the cross-section, we will find the area of the circle of the same radius as that of the sphere by using its formula.
Formula used:
Area of the circle
Complete step by step solution:
Here we need to find the area of the cross-section of the sphere of a given radius.
Here the cross-section of the sphere which is formed by a plane intersecting the sphere at an equator will be a circle of the same radius as that of the sphere and the given radius of the sphere is 11 inches.
So we will draw the diagram for the same.
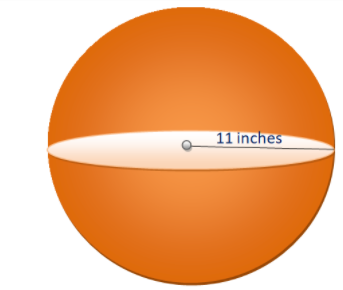
So to find the area of the cross-section, we will find the area of the circle of the same radius as that of the sphere.
We know that the formula of the area of a circle is equal to
Therefore, area of cross section
Now, we will substitute the value of pi. So, we get
On multiplying the numbers, we get
Note: A sphere is defined as a three-dimensional figure or a solid figure in which the distance of every point on its surface from its center is equidistant. However, a circle is defined as a two-dimensional figure or a plane figure in which the distance of every point on its circumference from its center is equidistant. Half of the sphere is called a hemisphere whereas half of the circle is called a semicircle.
Formula used:
Area of the circle
Complete step by step solution:
Here we need to find the area of the cross-section of the sphere of a given radius.
Here the cross-section of the sphere which is formed by a plane intersecting the sphere at an equator will be a circle of the same radius as that of the sphere and the given radius of the sphere is 11 inches.
So we will draw the diagram for the same.

So to find the area of the cross-section, we will find the area of the circle of the same radius as that of the sphere.
We know that the formula of the area of a circle is equal to
Therefore, area of cross section
Now, we will substitute the value of pi. So, we get
On multiplying the numbers, we get
Note: A sphere is defined as a three-dimensional figure or a solid figure in which the distance of every point on its surface from its center is equidistant. However, a circle is defined as a two-dimensional figure or a plane figure in which the distance of every point on its circumference from its center is equidistant. Half of the sphere is called a hemisphere whereas half of the circle is called a semicircle.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




