Question

Find the area of the Quadrilateral ABCD whose vertices are A ( -3, -1), B (-2, -4), C (4, -1) and D (3,4)

Answer 1

Hint- Proceed the solution of this question by dividing the quadrilateral in two triangles (using either of the diagonals), calculate the (positive value of) the areas of each triangle, and add these values to get the total area of the quadrilateral.

Complete step-by-step answer:

Let the vertices of the quadrilateral be A (-3, -1), B (-2, -4), C (4, -1) and D (3, 4).
Let AC be the diagonal of quadrilateral ABCD.
In the following figure, quadrilateral ABCD has been divided into ΔABC and ΔADC

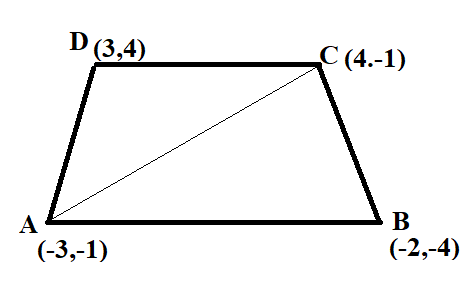
Therefore,
Area of quadrilateral ABCD = Area of Δ ABC + Area of Δ ADC ………… (1)

We know that,
Area of triangle = \[\dfrac{1}{2}\left| {[{{\text{x}}_1}({{\text{y}}_2} - {{\text{y}}_3}) + {{\text{x}}_2}({{\text{y}}_3} - {{\text{y}}_1}) + {{\text{x}}_3}({{\text{y}}_1} - {{\text{y}}_2})]} \right|\]

Thus,
Let triangle be ABC, where A= (−3, −1), B= (−2, −4), C= (4, -1)

Let the coordinates of point A $\left( {{{\text{x}}_1},{{\text{y}}_1}} \right)$ ,B $\left( {{{\text{x}}_2},{{\text{y}}_2}} \right)$ and C $\left( {{{\text{x}}_3},{{\text{y}}_3}} \right)$
So, \[{{\text{x}}_1} = - 3,{{\text{y}}_1} = - 1,{{\text{x}}_2} = - 2,{{\text{y}}_2} = - 4,{{\text{x}}_3} = 4,{{\text{y}}_3} = - 1\]

So using Above formula,

 Area of triangle Δ ABC=
⇒\[\dfrac{1}{2}\left| {[( - 3) \times \left( {( - 4) - ( - 1)} \right) + ( - 2) \times \left( {( - 1) - ( - 1)} \right) + (4) \times \left( {( - 1) - ( - 4)} \right)]} \right|\]

On further solving
⇒\[\dfrac{1}{2}\left| {[( - 3) \times \left( {( - 4) + 1} \right) + ( - 2) \times \left( {( - 1) + 1} \right) + (4) \times \left( {( - 1) + 4} \right)]} \right|\]
⇒\[\dfrac{1}{2}\left| {[( - 3) \times \left( { - 3} \right) + ( - 2) \times \left( {(0} \right) + (4) \times \left( 3 \right)]} \right|\]
⇒\[\dfrac{1}{2}\left| {[9 + 0 + 12]} \right|\]
⇒\[\dfrac{1}{2}\left| {[21]} \right| = \dfrac{{21}}{2}\] Sq. units

Now let triangle be ADC, where A= (−3, −1), D= (3, 4), C= (4, -1)

Let the coordinates of point A $\left( {{{\text{x}}_1},{{\text{y}}_1}} \right)$ ,D $\left( {{{\text{x}}_2},{{\text{y}}_2}} \right)$ and C $\left( {{{\text{x}}_3},{{\text{y}}_3}} \right)$
So, \[{{\text{x}}_1} = - 3,{{\text{y}}_1} = - 1,{{\text{x}}_2} = 3,{{\text{y}}_2} = 4,{{\text{x}}_3} = 4,{{\text{y}}_3} = - 1\]

So using Above formula,

 Area of triangle Δ ADC=
⇒\[\dfrac{1}{2}\left| {[( - 3) \times \left( {(4) - ( - 1)} \right) + (3) \times \left( {( - 1) - ( - 1)} \right) + (4) \times \left( {( - 1) - (4)} \right)]} \right|\]

On further solving
⇒\[\dfrac{1}{2}\left| {[( - 3) \times \left( {(4) + 1} \right) + (3) \times \left( {( - 1) + 1} \right) + (4) \times \left( {( - 1) - 4} \right)]} \right|\]
⇒\[\dfrac{1}{2}\left| {[( - 3) \times \left( 5 \right) + (3) \times \left( 0 \right) + (4) \times \left( { - 5} \right)]} \right|\]
⇒\[\dfrac{1}{2}\left| {[ - 15 + 0 - 20]} \right|\]
⇒\[\dfrac{1}{2}\left| {[ - 35]} \right| = \left| {\dfrac{{ - 35}}{2}} \right| = \dfrac{{35}}{2}\] Sq. units

Thus,
Substitute these values in equation (1), we have
Area of quadrilateral ABCD = Area of Δ ABC + Area of Δ ADC

Area of quadrilateral ABCD = \[\dfrac{{21}}{2}{\text{ + }}\dfrac{{35}}{2} = \dfrac{{56}}{2} = 28\] Sq. units

Hence, the area of the quadrilateral is 28 square units.

Note- In this particular question, sometimes it’s difficult to remember the formula of Area of triangle
which states above so there is also one other form of Area of triangle
Formula in determinant form which is as Area of triangle = $\left| {\dfrac{1}{2}\left| {\begin{array}{*{20}{c}}
  {{{\text{x}}_1}}&{{{\text{y}}_1}}&1 \\
  {{{\text{x}}_2}}&{{{\text{y}}_2}}&1 \\
  {{{\text{x}}_3}}&{{{\text{y}}_3}}&1
\end{array}} \right|} \right|$
We can also find the area of a triangle using this formula.