
Find the area of the sector of a circle when the angle of the sector is
A) 35
B) 45
C) 55
D) 65
Answer
478.8k+ views
1 likes
Hint:
Here we will use the formula for the area of a sector of a circle. We will substitute the values of
Formulas used:
We will use the following formulas:
The diameter of a circle is 2 times its radius:
Area of a sector of a circle with radius
Complete step by step solution:
First we will draw the circle showing the sector.
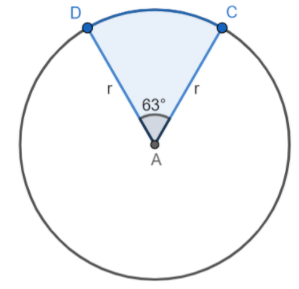
We will find the radius of the circle.
Substituting 20 for diameter in
The radius of the circle is 10 cm.
We will now find the area of the given sector.
By substituting
Simplifying the expression, we get
Note:
If we are unable to recall the formula for the area of a sector, we can derive it using the unitary method.
We know that the area of a circle is
The area of a sector with an angle of 360 degrees is
The area of a sector with an angle of 1 degree will be
The area of a sector with an angle of
Here we will use the formula for the area of a sector of a circle. We will substitute the values of
Formulas used:
We will use the following formulas:
The diameter of a circle is 2 times its radius:
Area of a sector of a circle with radius
Complete step by step solution:
First we will draw the circle showing the sector.

We will find the radius of the circle.
Substituting 20 for diameter in
The radius of the circle is 10 cm.
We will now find the area of the given sector.
By substituting
Simplifying the expression, we get
Note:
If we are unable to recall the formula for the area of a sector, we can derive it using the unitary method.
We know that the area of a circle is
The area of a sector with an angle of 360 degrees is
The area of a sector with an angle of 1 degree will be
The area of a sector with an angle of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




