
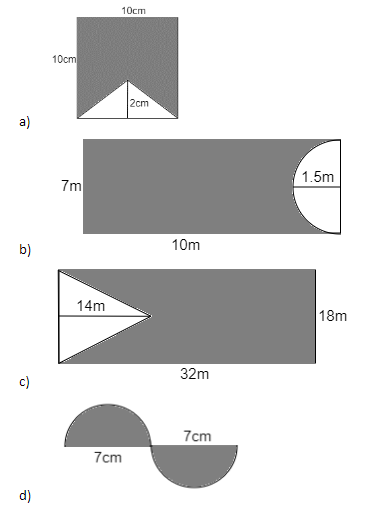
Find the area of the shaded portion.

Answer
497.4k+ views
Hint: In part (a), find the area of the square by the formula
Complete step-by-step answer:
Here side = 10 cm,
Square the term on the right side,
The area of the triangle is given by,
Here, the base of the triangle,
Cancel out the common factors,
Thus, the area of the shaded region is,
Substitute the values,
Subtract the terms,
Hence, the area of the shaded region is
Here the length of the rectangle,
Multiply the term on the right side,
The area of the semi-circle is given by,
Here, the radius of the circle,
Cancel out the common factors and square the terms,
Multiply the terms,
Round-off to two decimals,
Thus, the area of the shaded region is,
Substitute the values,
Subtract the terms,
Hence, the area of the shaded region is
Here the length of the rectangle,
Multiply the term on the right side,
The area of the triangle is given by,
Here, the base of the triangle,
Cancel out the common factors,
Multiply the terms,
Thus, the area of the shaded region is,
Substitute the values,
Subtract the terms,
Hence, the area of the shaded region is
The area of the circle is given by,
Here, the radius of the circle,
Square the term,
Cancel out the common factors and multiply the terms,
Hence, the area of the shaded region is
Note: Mensuration 2D mainly deals with problems on perimeter and area. The shape is two dimensional, such as triangle, square, rectangle, circle, parallelogram, etc.
Perimeter: The length of the boundary of a 2D figure is called the perimeter.
Area: The region enclosed by the 2D figure is called the area.
Pythagoras Theorem: In a right-angled triangle,
Complete step-by-step answer:
Here side = 10 cm,
Square the term on the right side,
The area of the triangle is given by,
Here, the base of the triangle,
Cancel out the common factors,
Thus, the area of the shaded region is,
Substitute the values,
Subtract the terms,
Hence, the area of the shaded region is
Here the length of the rectangle,
Multiply the term on the right side,
The area of the semi-circle is given by,
Here, the radius of the circle,
Cancel out the common factors and square the terms,
Multiply the terms,
Round-off to two decimals,
Thus, the area of the shaded region is,
Substitute the values,
Subtract the terms,
Hence, the area of the shaded region is
Here the length of the rectangle,
Multiply the term on the right side,
The area of the triangle is given by,
Here, the base of the triangle,
Cancel out the common factors,
Multiply the terms,
Thus, the area of the shaded region is,
Substitute the values,
Subtract the terms,
Hence, the area of the shaded region is
The area of the circle is given by,
Here, the radius of the circle,
Square the term,
Cancel out the common factors and multiply the terms,
Hence, the area of the shaded region is
Note: Mensuration 2D mainly deals with problems on perimeter and area. The shape is two dimensional, such as triangle, square, rectangle, circle, parallelogram, etc.
Perimeter: The length of the boundary of a 2D figure is called the perimeter.
Area: The region enclosed by the 2D figure is called the area.
Pythagoras Theorem: In a right-angled triangle,
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Differentiate between the Western and the Eastern class 9 social science CBSE




