Question

How do you find the center and vertices of the ellipse \[4{{x}^{2}}+{{y}^{2}}=1\]?

Answer 1

Hint: The standard form of equation of ellipse is \[\dfrac{{{x}^{2}}}{{{a}^{2}}}+\dfrac{{{y}^{2}}}{{{b}^{2}}}=1\]. And a, b are any real numbers. As we can see that here the coefficients of the square terms are in the denominator, so to find the center and vertices using the equation, we first have to take the coefficient of the \[{{x}^{2}}\] in the denominator. The vertices are similar to the intercepts of the ellipse. The centre of the ellipse with the equation \[\dfrac{{{x}^{2}}}{{{a}^{2}}}+\dfrac{{{y}^{2}}}{{{b}^{2}}}=1\] is \[x=0\And y=0\].

Complete step by step solution:
We are given the equation of the ellipse as \[4{{x}^{2}}+{{y}^{2}}=1\]. Comparing it with the standard form of the equation \[\dfrac{{{x}^{2}}}{{{a}^{2}}}+\dfrac{{{y}^{2}}}{{{b}^{2}}}=1\], we get \[{{a}^{2}}=\dfrac{1}{4}\And {{b}^{2}}=1\]. Taking the square root of both these equations to find the values, we get \[a=\dfrac{1}{2}\And b=1\].
We know that to find the center of the ellipse, we have to equate the x and y with zero. Thus, we get coordinates of centre as \[(0,0)\].
The vertices are the intercepts of the ellipse; thus, we can find them by substituting x and y to be zero separately.
Substituting \[x=0\], we get
\[\begin{align}
  & \Rightarrow 4{{(0)}^{2}}+{{y}^{2}}=1 \\
 & \Rightarrow {{y}^{2}}=1 \\
\end{align}\]
Solving the above equation, we get \[y=\pm 1\]. Thus, the coordinates of the two vertices are \[\left( 0,1 \right)\And \left( 0,-1 \right)\].
Substituting \[y=0\],
\[\begin{align}
  & \Rightarrow 4{{x}^{2}}+{{0}^{2}}=1 \\
 & \Rightarrow 4{{x}^{2}}=1 \\
\end{align}\]
Solving the above equation, we get \[x=\pm \dfrac{1}{2}\]. Thus, the coordinates of the other two vertices are \[\left( \dfrac{1}{2},0 \right)\And \left( -\dfrac{1}{2},0 \right)\].
We can graph the ellipse as,

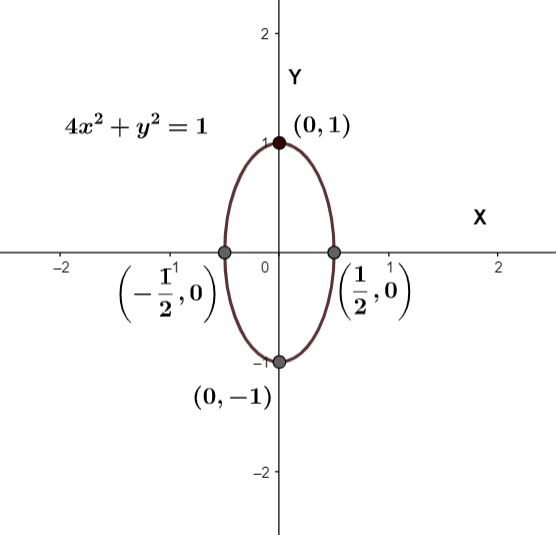
Note: Here, as the coefficient of the \[{{x}^{2}}\] was not of the form of \[\dfrac{1}{{{a}^{2}}}\]. We have to equate this with the given coefficient, to find the value of the a. we can do this as, \[\dfrac{1}{{{a}^{2}}}=4\]. Thus, on solving this equation, we get \[a=\dfrac{1}{2}\].