
How do you find the diameter of a hole?
Answer
449.7k+ views
Hint: In this question we have been asked to find the diameter of a hole. We will look at the various characteristics related to a hole like the radius, area and circumference and look at how we can find the value of the diameter by rearranging the terms in the formula of circumference and area. we will use the formula
Complete step-by-step answer:
We know that a hole is a hollow place found in a rigid body or a rigid surface. The diameter is a property of a circle which tells us the length of the line which passes through the center of the circle and touches the two points on the circle on opposite sides.
A hole can be represented in the form of a circle as:
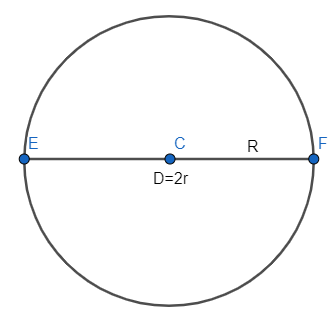
In the figure line segment
We know that
In the case we know the circumference of a hole, we can rearrange the terms in the formula of circumference.
The formula of circumference is
With this formula, we can find the diameter of the circle.
Note: It is to be remembered that a hole is also possible in a three-dimensional surface. When a hole is present in three dimensions, the hole represents a cylinder. It is also not always the case that a hole will be circular in shape. There could be holes of vague shapes for which the formulas used in the above question cannot be used.
Complete step-by-step answer:
We know that a hole is a hollow place found in a rigid body or a rigid surface. The diameter is a property of a circle which tells us the length of the line which passes through the center of the circle and touches the two points on the circle on opposite sides.
A hole can be represented in the form of a circle as:

In the figure line segment
We know that
In the case we know the circumference of a hole, we can rearrange the terms in the formula of circumference.
The formula of circumference is
With this formula, we can find the diameter of the circle.
Note: It is to be remembered that a hole is also possible in a three-dimensional surface. When a hole is present in three dimensions, the hole represents a cylinder. It is also not always the case that a hole will be circular in shape. There could be holes of vague shapes for which the formulas used in the above question cannot be used.
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

What does R mean in math class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





