Question

How do you find the inflection points of the graph of the function: $f(x) = 12{x^5} + 45{x^4} - 80{x^3} + 6$

Answer 1

Hint: Second derivation of any kind of graph function shows inflection point.
When we do a second derivation of the graph function to see for the change in the concavity nature of the graph like change from concave up to concave down or vice versa. The point at which the change starts is called the inflection point.

The inflection points are given when the second derivative is equalled to zero. Because the second derivative indicates the change in the concavity of the graph function in the question.

Complete step by step answer:
From the question, we can see that the given equation is
$f(x) = 12{x^5} + 45{x^4} - 80{x^3} + 6$
So, we first begin by doing the first derivative of the function, and then proceed to the second derivation.
FIRST DERIVATION: -
To find the inflection and turning points of $f(x)$, we follow the usual procedure and set \[f'\left( x \right) = 0\] .
\[f(x) = 12{x^5} + 45{x^4} - 80{x^3} + 6\]
So, on derivation we get;
\[f'(x) = 60{x^4} + 180{x^3} - 240{x^2}\]
Now this is a quartic equation. A full "solution by radicals" of the quartic is known, but it is long and complex (and painful to perform!). Fortunately, we can factorise this equation:
\[f'(x) = 60{x^2}({x^2} + 3x - 4) = 60{x^2}(x + 4)(x - 1).\]
To categorise these, consider the second derivative:
\[f''(x) = 240{x^3} + 540{x^2} - 480x = 60x(4{x^2} + 9x - 8)\]
\[
  f''\left( { - 4} \right) = - 4800 < 0 \\
  f''\left( 0 \right) = 0 \\
  f''\left( 1 \right) = 300 > 0 \\
\]
So, -4 is a maximum of the function and +1 is a minimum. To categorise 0, we need to take another derivative.
\[
  f'''(x) = 720{x^2} + 1080x - 480,{\text{ }} \\
  so\;f'''(0) = - 480 \ne 0 \\
 \]
Thus 0 is a point of inflection.
Below is the graphical representation of the given equation with the inflection points marked.

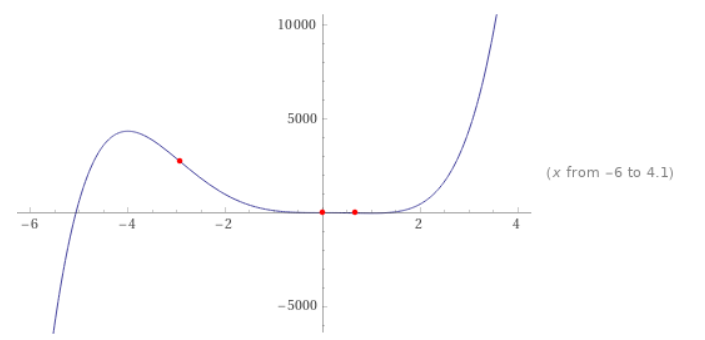
Note: The inflection is the point at which the nature of the concave graph changes from one to another (concave up/concave down) rather than the start points or the end point of the curve of the graph of the given function equation.