Question

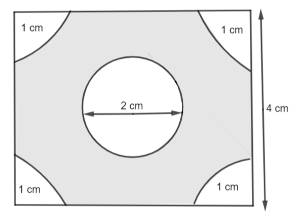
Find the perimeter and the area of the shaded region shown in the figure. The four corners are the circle quadrants and at the centre, there is a circle. \[\left[ \text{Take }\pi =3.14 \right]\]

(a) Perimeter = 20.56 cm
\[\left( \text{b} \right)\text{ Area}=9.72c{{m}^{2}}\]
\[\left( \text{c} \right)\text{ Area}=20.56c{{m}^{2}}\]
(d) Perimeter = 9.72 cm

Answer 1

Hint: First of all find the area of the shaded portion by subtracting the area of the unshaded portion that is the circle and 4 quadrants from the total area that is the area of the square. Find the perimeter by adding the length of the total outline of the given shaded region which includes 4 lengths, 4 arcs of the quadrants and the circumference of the centre circle. We know that the formula for area of circle \[\pi {{\left( r \right)}^{2}}\] , so for quadrant it will be one-fourth of this and area of square is \[{{\left( \text{side} \right)}^{2}}.\] Then, we will add up all the side lengths to get the perimeter.

Complete step-by-step answer:
In this question, we have to find the perimeter and the area of the shaded region in the given figure

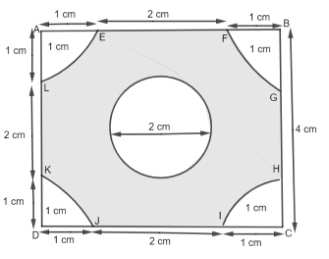
Let us first name the given figure as AEFBGHCIJDKL as shown in the above figure. Now, first of all, let us find the area of the figure that is the area of the square ABCD.
We know that the area of the square is \[{{\left( \text{side} \right)}^{2}}.\] We are given that the side of the square is 4 cm. So, we get,
\[\text{Area of square }ABCD={{\left( 4cm \right)}^{2}}=16c{{m}^{2}}.......\left( i \right)\]
Now, let us find the area of the unshaded region. From the figure, we get,
\[\begin{align}
  & \text{Area of unshaded region}=4\times \left( \text{Area of the circular quadrant at the corner} \right) \\
 & +\text{Area of the circle at the center}.......\left( ii \right) \\
\end{align}\]
Let us find the area of the circular quadrant. We know that the area of the circle is \[\pi {{\left( r \right)}^{2}}\] where r is the radius of the circle. As the quadrant is one – fourth part of the circle, so we get,
\[\text{Area of the circular quadrant}=\dfrac{\pi {{r}^{2}}}{4}\]
We are given that r = 1 cm. So, we get,
\[\text{Area of the circular quadrant}=\dfrac{\pi {{\left( 1cm \right)}^{2}}}{4}=\dfrac{\pi }{4}c{{m}^{2}}......\left( iii \right)\]
Now, let us find the area of the circle at the centre. We are given that its diameter = 2 cm. So, we get its radius as
\[\text{Radius}=\dfrac{\text{Diameter}}{2}=\dfrac{2cm}{2}=1cm\]
Therefore, the area of the circle at the centre is
\[=\pi {{r}^{2}}\]
\[\Rightarrow \pi {{\left( 1cm \right)}^{2}}\]
\[\Rightarrow \pi c{{m}^{2}}......\left( iv \right)\]
Now, by substituting the area of the quadrant and the circle from equation (iii) and (iv), respectively in equation (ii), we get,
\[\text{Area of unshaded region}=4\left( \dfrac{\pi }{4} \right)+\pi \]
\[\text{Area of unshaded region}=2\pi c{{m}^{2}}........\left( v \right)\]
From the figure, we get,
Area of shaded region = Total area of the square – Area of the unshaded region
By substituting the value of the area of the square and the unshaded region from equation (i) and (v) respectively, we get,
\[\text{Area of shaded region}=16c{{m}^{2}}-2\pi c{{m}^{2}}\]
By using \[\pi =3.14,\] we get,
\[\Rightarrow \text{Area of shaded region}=16c{{m}^{2}}-2\left( 3.14 \right)c{{m}^{2}}\]
\[\Rightarrow \text{Area of shaded region}=16c{{m}^{2}}-6.28c{{m}^{2}}\]
\[\Rightarrow \text{Area of shaded region}=9.72c{{m}^{2}}\]
Now, let us find the perimeter of the shaded region. We know the perimeter is basically the length of the outline of a region.
So, from the figure, we get,
Perimeter of shaded region = EF + GH + IJ + KL + Arc FG + Arc HI + Arc JK + Arc LE + Circumference of the circle at the centre.
We know that EF = GH = IJ = KL = 2 cm and Arc FG = Arc HI = Arc JK = Arc LE. So, we get,
\[\text{Perimeter of shaded region}=2cm+2cm+2cm+2cm+4\times \left( \text{Arc FG} \right)+\text{Circumference of circle at the center}\]
\[\text{Perimeter of shaded region}=8cm+4\times \left( \text{Arc FG} \right)+\text{Circumference of circle at the center}.......\left( vi \right)\]
We know that the perimeter of the circular quadrant is one – fourth of the circumference of the circle that is \[2\pi r.\] So, we get,
\[\text{Length of Arc FG}=\dfrac{2\pi r}{4}\]
\[\Rightarrow \text{Length of Arc FG}=\dfrac{\pi r}{2}\]
By using \[\pi =3.14\] and r = 1 cm, we get,
\[\Rightarrow \text{Length of Arc FG}=\dfrac{3.14\times 1}{2}\]
\[\Rightarrow \text{Length of Arc FG}=1.57cm......\left( vii \right)\]
Also, we get the circumference of the circle at the centre as \[2\pi r.\] By using r = 1 cm, we get,
Circumference of the circle at the centre \[=2\left( 3.14 \right)\left( 1 \right)=6.28cm.......\left( viii \right)\]
By substituting the length of the arc FG and circumference of the circle at the centre from equation (vii) and (viii) in equation (vi), we get,
Perimeter of shaded region = 8 cm + 4 (1.57 cm) + 6.28 cm
\[=8+6.28+6.28\]
Therefore, the perimeter of the shaded region = 20.56 cm.
So, we get the area as \[9.72c{{m}^{2}}\] and the perimeter as 20.56 cm.
Hence, option (a) and (b) are the right answers.

Note: In this question, while calculating the perimeter, students often forget to add the circumference of the circle at the centre but it should be added as it is also outlining the shaded area. Also, students often make this mistake of taking the radius of the centre circle as 2 cm which is actually the diameter. So, this must be taken care of while calculating its area as well as circumference. Also, take care that the unit of the area would be \[{{\left( cm \right)}^{2}}\] while for perimeter it would be (cm) only.