
Find the square root of the following decimal numbers:
Find the square root of the following decimal numbers:
A.2.56
B.7.29
C.51.84
D.42.25
E.31.36
Answer
515.7k+ views
Hint: Mark the period in both integral and decimal parts in a pair. After that find the square root by long division method and put the decimal point on the square root.
Complete step-by-step answer:
Let us take the first decimal number 2.56. Let us divide it with 1.
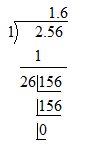
We know,
The square root of 2.56 = 1.6
So step 1 is to make the number of decimal places even by affixing a zero on the extreme right of the decimal part if it’s not even. As step 2, in the integral part, mark the period,
Now let us find the square root of 7.29
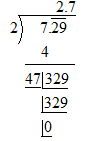
We know,
Square root of 51.84
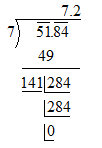
Now let’s get the square root of 42.25
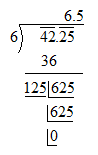
Thus square root of 42.25 = 6.5
Square root of 31.36
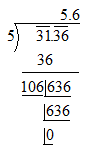
Thus we got the square root of the following decimal numbers as,
2.56 = 1.6
7.29 = 2.7
51.84 = 7.2
42.25 = 6.5
31.36 = 5.6
Note: Remember that the division of the integral part must be by a perfect square i.e. (2
Complete step-by-step answer:
Let us take the first decimal number 2.56. Let us divide it with 1.
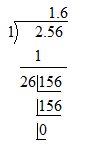
We know,
The square root of 2.56 = 1.6
So step 1 is to make the number of decimal places even by affixing a zero on the extreme right of the decimal part if it’s not even. As step 2, in the integral part, mark the period,
Now let us find the square root of 7.29
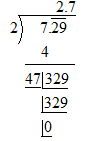
We know,
Square root of 51.84
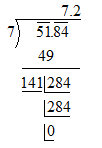
Now let’s get the square root of 42.25
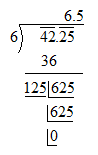
Thus square root of 42.25 = 6.5
Square root of 31.36
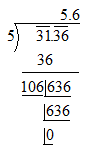
Thus we got the square root of the following decimal numbers as,
2.56 = 1.6
7.29 = 2.7
51.84 = 7.2
42.25 = 6.5
31.36 = 5.6
Note: Remember that the division of the integral part must be by a perfect square i.e. (2
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




