Question

Find the value of $\dfrac{{LK}}{{KJ}}$ from the given figure.

.

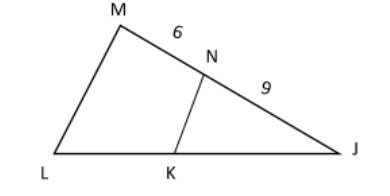Answer 1

Hint: To solve this and find the ratio,first we will try to find the value of MK, LK, KN, MN and the perimeter of quadrilateral MNKL.


Complete step by step solution:
 Now we have given $MN = 6,NJ = 9$ and $ML\parallel NK$
Triangle Proportionality Theorem:
If a line is parallel to one side of a triangle and intersects the other two sides, then it divides the sides into segments of proportional lengths.
Use the Triangle Proportionality Theorem, we obtain
$\dfrac{{MN}}{{NJ}} = \dfrac{{LK}}{{KJ}}.......\left( i \right)$
Here,$MN = 6,NJ = 9$
Then we will substitute the value of $MN and NJ$ in equation$\left( i \right)$, we have
\[
  \dfrac{{MN}}{{NJ}} = \dfrac{{LK}}{{KJ}} \\
  \dfrac{6}{9} = \dfrac{{LK}}{{KJ}} \\
   \Rightarrow \dfrac{{LK}}{{KJ}} = \dfrac{6}{9} \\
 \]


Note: Students must keep in mind that while you use the basic Proportionality Theorem their one pair should be parallel. Then you apply the result of the basic Proportionality Theorem otherwise not use the theorem.