
Find the value of the unknown exterior angle
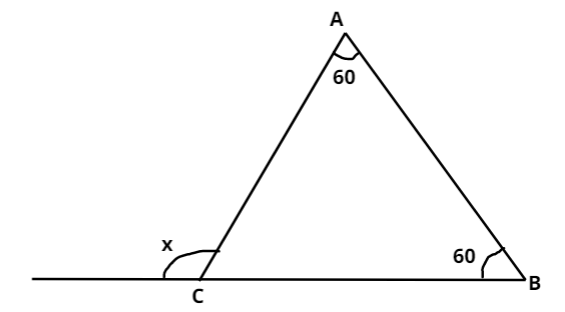

Answer
491.4k+ views
Hint: First of all find the third unknown angle of the triangle by using the condition that the sum of all internal angles of a triangle is
Complete step-by-step answer:
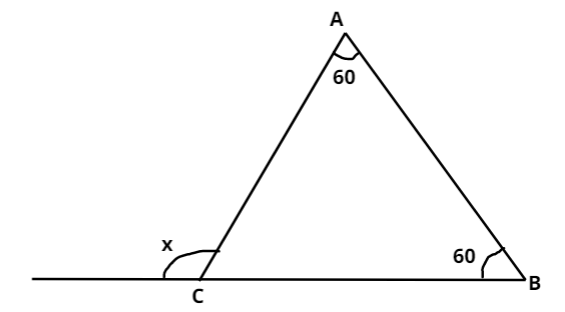
Let the vertices of the triangle are A, B and C as shown in the above figure.
Two of the triangle’s angles are already given in the question. So we have:
According to the triangle's law, we know that the sum of all the internal angles of a triangle is
Putting
Thus the third internal angle of the triangle is also
Further, from the figure, we can say that the sum of the internal angle C and the external angle
Putting the value of
Therefore, the value of exterior angle
Note: When all the angles of a triangle are equal then the triangle is called equilateral triangle. The measure of each angle in such a triangle is
When only two angles of a triangle are the same then the triangle is called isosceles triangle. And when all the angles are different then it is called scalene triangle.
Complete step-by-step answer:

Let the vertices of the triangle are A, B and C as shown in the above figure.
Two of the triangle’s angles are already given in the question. So we have:
According to the triangle's law, we know that the sum of all the internal angles of a triangle is
Putting
Thus the third internal angle of the triangle is also
Further, from the figure, we can say that the sum of the internal angle C and the external angle
Putting the value of
Therefore, the value of exterior angle
Note: When all the angles of a triangle are equal then the triangle is called equilateral triangle. The measure of each angle in such a triangle is
When only two angles of a triangle are the same then the triangle is called isosceles triangle. And when all the angles are different then it is called scalene triangle.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




