Question

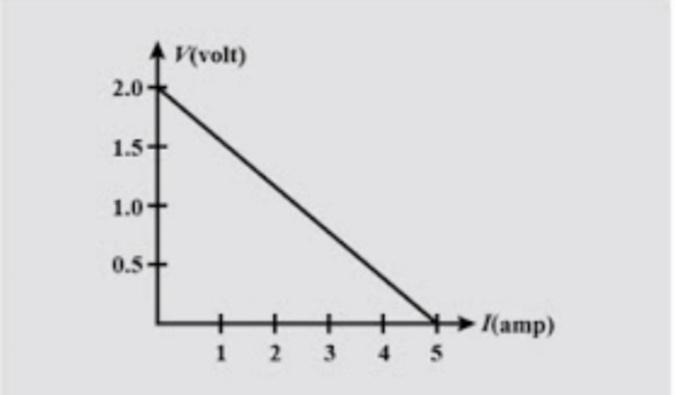
For a cell, a graph is plotted between the potential difference V across the terminals of the cell and the current I drawn from the cell (see figure). The emf and the internal resistance of the cell are E and r, respectively. Then:

A. E= 2V, r = 0.5Ω
B. E= 2V, r = 0.4Ω
C. E > 2V, r = 0.5Ω
D. E > 2V, r = 0.4Ω

Answer 1

Hint: Use minimum and maximum values of potential difference and current in the formula of emf of the cell. By using the peak values of current and emf of the cell from the graph we can easily determine the emf and internal resistance of the cell.

Complete step-by-step answer:
We know that when there is current present in the device or the electrical circuit and there's a voltage drop in source voltage or source battery is internal resistance.
It is caused due to electrolytic material in batteries or other voltage sources.
As we know that, the emf of a cell having internal resistance r is given by
\[{\text{V = E - Ir}}\]
Where,
V= potential difference
E= e.m.f.
I=current
r= internal resistance
Now, we put values from graph in the above equation in order to find emf of cell and resistance
Take \[{\text{V}}\]= 2V and I= 0 from the graph
\[{\text{V = E - Ir}}\]
On putting the values we get

${\text{2 = E - }}\left( {\text{0}} \right){\text{r}}$
On solving we get
${\text{E = 2V}}$
Here, the current is zero so the maximum potential difference is equals to emf. So, the value of emf is 2V.
Now, put V=0, E= 2V and I=5A in the formula of emf of cell we have
\[{\text{V = E - Ir}}\]
${\text{0 = 2 - 5r}}$
${\text{r = }}\dfrac{{\text{2}}}{{\text{5}}}{\text{ = 0}}{\text{.4 }}\Omega$
So, the value of E= 2V and r= 0.4Ω
$\therefore $Option (B) is correct.

Note: Be careful while using the concept of emf and potential difference in case of maximum potential difference. The smaller the internal resistance for a given emf, the more current and the more power the source can supply. Any voltage source has an emf related to its source of potential difference, and an internal resistance r related to its construction.