
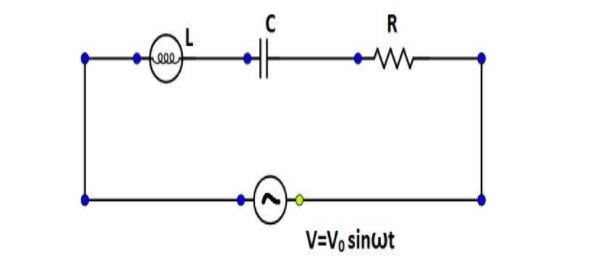
For the LCR circuit, shown here, the current is observed to lead the applied voltage. An additional capacitor $C'$, when joined with the capacitor $C$ present in the circuit, makes the power factor of the circuit unity. The capacitor $C'$ must have been connected in:
(A) $\dfrac{{1 - {\omega ^2}LC}}{{{\omega ^2}L}}$parallel with $C$
(B) $\dfrac{{1 - {\omega ^2}LC}}{{{\omega ^2}L}}$series with $C$
(C) $\dfrac{C}{{\left( {{\omega ^2}LC - 1} \right)}}$parallel with $C$
(D) $\dfrac{C}{{\left( {{\omega ^2}LC - 1} \right)}}$series with $C$
Answer
117.9k+ views
Hint: Given that the power factor of the circuit is unity. The difference of impedance reactance and capacitive reactance is zero. So we need to compute their values and solve the equation to find the answer.
Formula Used: The formulae used in the solution are given here.
The impedance of circuit is given by $Z = \sqrt {{R^2} + {{\left( {\omega L - \dfrac{1}{{\omega C}}} \right)}^2}} $ where $R$ is the resistance and $C$ is the capacitance and $\omega = 2\pi f$ where $f$ is the frequency.
${X_L}$ is impedance reactance and ${X_C}$ is capacitive reactance.
Complete Step by Step Solution: In general power is the capacity to do work. In the electrical domain, electrical power is the amount of electrical energy that can be transferred to some other form (heat, light etc.) per unit time. Mathematically it is the product of voltage drop across the element and current flowing through it. Considering first the DC circuits, having only DC voltage sources, the inductors and capacitors behave as short circuits and open circuits respectively in steady state.
Now coming to AC circuit, here both inductor and capacitor offer a certain amount of impedance given by:
${X_L} = 2\pi fL$ and ${X_C} = \dfrac{1}{{2\pi fC}}$.
The inductor of impedance $L$ stores electrical energy in the form of magnetic energy and capacitor of capacitance $C$ stores electrical energy in the form of electrostatic energy.
Neither of them dissipates it. Further, there is a phase shift between voltage and current.
The cosine of this phase difference is called electrical power factor. This factor ($ - 1 < \cos \varphi < 1$ ) represents the fraction of the total power that is used to do the useful work. The other fraction of electrical power is stored in the form of magnetic energy or electrostatic energy in the inductor and capacitor respectively.
Given that, the current is observed to lead the applied voltage in the LCR circuit. An additional capacitor $C'$, when joined with the capacitor $C$ present in the circuit, makes the power factor of the circuit unity.
Thus, $\cos \varphi = 1$.
$\cos \varphi = \dfrac{R}{{\sqrt {{R^2}\left[ {\omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}}} \right]} }} = 1$.
On solving the equation above, we get,
$ \Rightarrow \omega L = \dfrac{1}{{\omega \left( {C + C'} \right)}}$
The capacitor $C'$ must have magnitude:
$C' = \dfrac{{1 - {\omega ^2}LC}}{{{\omega ^2}L}}$
Adding capacitor of capacitance C' in parallel of C, the reactance will be:
${X_L} - {X_C} = \omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}}$
Since, ${X_L} - {X_C} = 0$,
$\omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}} = 0$
$ \Rightarrow C' = \dfrac{1}{{{\omega ^2}L}} - C$
Connecting the capacitors in parallel, $C' = \dfrac{{1 - {\omega ^2}LC}}{{{\omega ^2}L}}$
Hence the correct answer is Option A.
Note: The impedance of circuit is given by $Z = \sqrt {{R^2} + {{\left( {\omega L - \dfrac{1}{{\omega C}}} \right)}^2}} $ and the current lag voltage by $\tan \varphi = \dfrac{{{X_L} - {X_C}}}{R} = \dfrac{{\omega L - \dfrac{1}{{\omega C}}}}{R}$
For the power factor to be one the current and voltage have to be in the same phase i.e. $\varphi $ has to be zero.
Adding capacitor of capacitance $C'$ in series of $C$, the reactance will be
${X_L} - {X_C} = \omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}}$
$ \Rightarrow \omega L - \dfrac{1}{{\omega \left( {\dfrac{{CC'}}{{C + C'}}} \right)}}$
Which gives us,
$ \Rightarrow {\omega ^2}LCC' = C + C'$
The value of $C'$ when connected in series will be,
Thus, $C' = \dfrac{C}{{{\omega ^2}LC - 1}}$.
Formula Used: The formulae used in the solution are given here.
The impedance of circuit is given by $Z = \sqrt {{R^2} + {{\left( {\omega L - \dfrac{1}{{\omega C}}} \right)}^2}} $ where $R$ is the resistance and $C$ is the capacitance and $\omega = 2\pi f$ where $f$ is the frequency.
${X_L}$ is impedance reactance and ${X_C}$ is capacitive reactance.
Complete Step by Step Solution: In general power is the capacity to do work. In the electrical domain, electrical power is the amount of electrical energy that can be transferred to some other form (heat, light etc.) per unit time. Mathematically it is the product of voltage drop across the element and current flowing through it. Considering first the DC circuits, having only DC voltage sources, the inductors and capacitors behave as short circuits and open circuits respectively in steady state.
Now coming to AC circuit, here both inductor and capacitor offer a certain amount of impedance given by:
${X_L} = 2\pi fL$ and ${X_C} = \dfrac{1}{{2\pi fC}}$.
The inductor of impedance $L$ stores electrical energy in the form of magnetic energy and capacitor of capacitance $C$ stores electrical energy in the form of electrostatic energy.
Neither of them dissipates it. Further, there is a phase shift between voltage and current.
The cosine of this phase difference is called electrical power factor. This factor ($ - 1 < \cos \varphi < 1$ ) represents the fraction of the total power that is used to do the useful work. The other fraction of electrical power is stored in the form of magnetic energy or electrostatic energy in the inductor and capacitor respectively.
Given that, the current is observed to lead the applied voltage in the LCR circuit. An additional capacitor $C'$, when joined with the capacitor $C$ present in the circuit, makes the power factor of the circuit unity.
Thus, $\cos \varphi = 1$.
$\cos \varphi = \dfrac{R}{{\sqrt {{R^2}\left[ {\omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}}} \right]} }} = 1$.
On solving the equation above, we get,
$ \Rightarrow \omega L = \dfrac{1}{{\omega \left( {C + C'} \right)}}$
The capacitor $C'$ must have magnitude:
$C' = \dfrac{{1 - {\omega ^2}LC}}{{{\omega ^2}L}}$
Adding capacitor of capacitance C' in parallel of C, the reactance will be:
${X_L} - {X_C} = \omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}}$
Since, ${X_L} - {X_C} = 0$,
$\omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}} = 0$
$ \Rightarrow C' = \dfrac{1}{{{\omega ^2}L}} - C$
Connecting the capacitors in parallel, $C' = \dfrac{{1 - {\omega ^2}LC}}{{{\omega ^2}L}}$
Hence the correct answer is Option A.
Note: The impedance of circuit is given by $Z = \sqrt {{R^2} + {{\left( {\omega L - \dfrac{1}{{\omega C}}} \right)}^2}} $ and the current lag voltage by $\tan \varphi = \dfrac{{{X_L} - {X_C}}}{R} = \dfrac{{\omega L - \dfrac{1}{{\omega C}}}}{R}$
For the power factor to be one the current and voltage have to be in the same phase i.e. $\varphi $ has to be zero.
Adding capacitor of capacitance $C'$ in series of $C$, the reactance will be
${X_L} - {X_C} = \omega L - \dfrac{1}{{\omega \left( {C + C'} \right)}}$
$ \Rightarrow \omega L - \dfrac{1}{{\omega \left( {\dfrac{{CC'}}{{C + C'}}} \right)}}$
Which gives us,
$ \Rightarrow {\omega ^2}LCC' = C + C'$
The value of $C'$ when connected in series will be,
Thus, $C' = \dfrac{C}{{{\omega ^2}LC - 1}}$.
Recently Updated Pages
Uniform Acceleration - Definition, Equation, Examples, and FAQs

Young's Double Slit Experiment Step by Step Derivation

How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025: Application Form (Out), Exam Dates (Released), Eligibility & More

JEE Main Login 2045: Step-by-Step Instructions and Details

JEE Main Chemistry Question Paper with Answer Keys and Solutions

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Dual Nature of Radiation and Matter Class 12 Notes CBSE Physics Chapter 11 (Free PDF Download)

Charging and Discharging of Capacitor

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

Physics Average Value and RMS Value JEE Main 2025

Inductive Effect and Acidic Strength - Types, Relation and Applications for JEE




