Question

For the principal value, evaluate the following
\[{{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)-2{{\sec }^{-1}}\left( 2\tan \dfrac{\pi }{6} \right)\]

Answer 1

Hint:First of all, substitute the value of \[\tan \dfrac{\pi }{6}\] from the trigonometric table. Now find the angles at which \[\sin \theta =\dfrac{\sqrt{3}}{2}\] and \[sec\theta =\dfrac{2}{\sqrt{3}}\] or the value of \[{{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)\] and \[se{{c}^{-1}}\left( \dfrac{2}{\sqrt{3}} \right)\] and substitute these in the given expression to get the required answer.

Complete step-by-step answer:
In this question, we have to find the principal value of the expression \[{{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)-2{{\sec }^{-1}}\left( 2\tan \dfrac{\pi }{6} \right)\].
First of all, let us consider the expression given in the question,
\[E={{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)-2{{\sec }^{-1}}\left( 2\tan \dfrac{\pi }{6} \right)....\left( i \right)\]
Now, let us draw the table for trigonometric ratios of general angles.

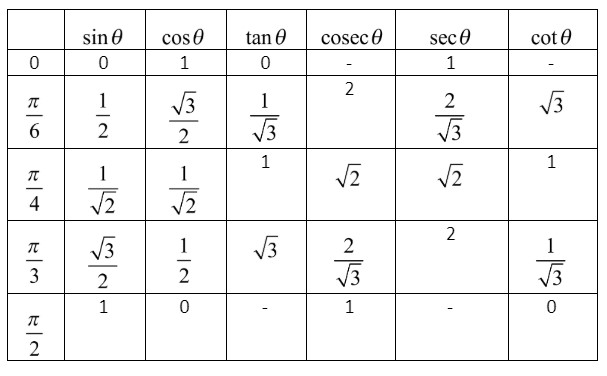
From the above table, we get, \[\tan \dfrac{\pi }{6}=\dfrac{1}{\sqrt{3}}\]. So, by substituting the value of \[\tan \dfrac{\pi }{6}\] in equation (i), we get,
\[E={{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)-2{{\sec }^{-1}}\left( 2.\dfrac{1}{\sqrt{3}} \right)\]
\[E={{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)-2{{\sec }^{-1}}\left( \dfrac{2}{\sqrt{3}} \right).....\left( ii \right)\]
Now we know that the range of principal value of \[{{\sin }^{-1}}\left( x \right)\] lies between \[\left[ \dfrac{-\pi }{2},\dfrac{\pi }{2} \right]\].
From the table of general trigonometric ratios, we get,
\[\sin \left( \dfrac{\pi }{3} \right)=\dfrac{\sqrt{3}}{2}\]
By taking \[{{\sin }^{-1}}\] on both the sides, we get,
\[{{\sin }^{-1}}\sin \left( \dfrac{\pi }{3} \right)={{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)\]
We know that for \[\dfrac{-\pi }{2}\le x\le \dfrac{\pi }{2},{{\sin }^{-1}}\sin \left( x \right)=x\]. So, we get,
\[\dfrac{\pi }{3}={{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)...\left( iii \right)\]
Now, we also know that the range of principal value of \[se{{c}^{-1}}x\] lies between \[\left[ 0,\pi \right]-\left\{ \dfrac{\pi }{2} \right\}\]
From the table of trigonometric ratios, we get,
\[sec\left( \dfrac{\pi }{6} \right)=\dfrac{2}{\sqrt{3}}\]
By taking \[se{{c}^{-1}}\] on both the sides, we get,
\[se{{c}^{-1}}\left( sec\left( \dfrac{\pi }{6} \right) \right)=se{{c}^{-1}}\left( \dfrac{2}{\sqrt{3}} \right)\]
We know that for \[\left[ 0\le x\le \pi \right]-\left\{ \dfrac{\pi }{2} \right\},se{{c}^{-1}}sec\left( x \right)=x\]. So, we get,
\[\dfrac{\pi }{6}=se{{c}^{-1}}\left( \dfrac{2}{\sqrt{3}} \right)....\left( iv \right)\]
So, by substituting the value of \[se{{c}^{-1}}\left( \dfrac{2}{\sqrt{3}} \right)\] from equation (iv) and \[{{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)\] from equation (iii) in equation (ii), we get,
\[E=\dfrac{\pi }{3}-2\left( \dfrac{\pi }{6} \right)\]
\[E=\dfrac{\pi }{3}-\dfrac{\pi }{3}\]
\[E=0\]
Hence, we get the value of \[{{\sin }^{-1}}\left( \dfrac{\sqrt{3}}{2} \right)-2{{\sec }^{-1}}\left( 2\tan \dfrac{\pi }{6} \right)\] as 0.

Note: In these types of questions, first of all, students must remember the trigonometric ratios at general angles like \[{{0}^{o}},{{30}^{o}},{{45}^{o}},{{60}^{o}},{{90}^{o}},\] etc. Also, students must note that \[{{\sin }^{-1}}\sin \left( x \right)=x\] and \[se{{c}^{-1}}\sec \left( x \right)=x\] are the only value for their respective ranges and not for other values of x. Students should not confuse between \[{{\sin }^{-1}}\sin x\] and \[\sin {{\sin }^{-1}}x\] and simplify for other trigonometric ratios as well.