
How do you graph
Answer
456.6k+ views
Hint: To solve this we need to give the values of ‘x’ and we can find the values of ‘y’. Otherwise we can find the coordinate of the given equation lying on the line of x- axis, we can find this by substituting the value of ‘y’ is equal to zero (x-intercept). Similarly we can find the coordinate of the equation lying on the line of y- axis, we can find this by substituting the value of ‘x’ equal to zero (y-intercept).
Complete step-by-step solution:
Given,
To find the x-intercept. That is the value of ‘x’ at
Divide the whole equation by 3 we have,
Thus we have a coordinate of the equation which lies on the line of x-axis. The coordinate is
To find the y-intercept. That is the value of ‘y’ at
Divide the whole equation by -4 we have,
Thus we have a coordinate of the equation which lies on the line of y-axis. The coordinate is
Let’s find one more coordinate by giving the random value of ‘x’.
Thus we have the coordinates
Let’s plot a graph for this coordinates,
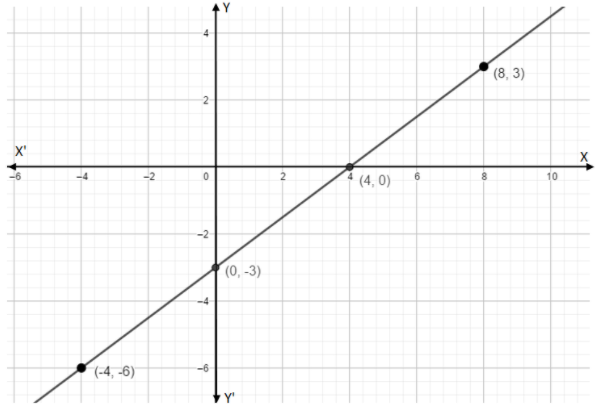
All we did was expand the line touching the coordinates
Without doing the calculation we found out two more coordinates. (See in the graph).
The coordinates are
Note: A graph shows the relation between two variable quantities, it contains two axes perpendicular to each other namely the x-axis and the y-axis. Each variable is measured along one of the axes. In the question, we are given one linear equation containing two variables namely x and y, x is measured along the x-axis and y is measured along the y-axis while tracing the given equations.
Complete step-by-step solution:
Given,
To find the x-intercept. That is the value of ‘x’ at
Divide the whole equation by 3 we have,
Thus we have a coordinate of the equation which lies on the line of x-axis. The coordinate is
To find the y-intercept. That is the value of ‘y’ at
Divide the whole equation by -4 we have,
Thus we have a coordinate of the equation which lies on the line of y-axis. The coordinate is
Let’s find one more coordinate by giving the random value of ‘x’.
Thus we have the coordinates
Let’s plot a graph for this coordinates,

All we did was expand the line touching the coordinates
Without doing the calculation we found out two more coordinates. (See in the graph).
The coordinates are
Note: A graph shows the relation between two variable quantities, it contains two axes perpendicular to each other namely the x-axis and the y-axis. Each variable is measured along one of the axes. In the question, we are given one linear equation containing two variables namely x and y, x is measured along the x-axis and y is measured along the y-axis while tracing the given equations.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

The first successful textile mill was established in class 9 social science CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

Name one staple crop of India and the regions where class 9 social science CBSE




