Answer
Verified430.8k+ views
Hint: For plotting a graph we need different x values as well as their corresponding y values. We know the equation $\dfrac{x}{a} + \dfrac{y}{b} = 1$ where $a,b$ are the x intercept and y intercept respectively. So by using this equation we can find the x intercept and y intercept and thereby plot the graph. After finding these values we just need to plot them on the XY plane.
Complete step by step solution:
Given
$4x - 3y = 12..........................\left( i \right)$
For graphing the given equation we need to find the x intercept and y intercept by using the
formula $\dfrac{x}{a} + \dfrac{y}{b} = 1$.
x intercept is the point where the graph touches X axis such that y=0 and y intercept is the point where the graph touches the Y axis such that x=0 .
So we know:
$\dfrac{x}{a} + \dfrac{y}{b} = 1...........................\left( {ii} \right)$
So we need to convert equation (i) in terms of (ii) and thereby find the values of
$a\,{\text{and}}\,b$ which are x intercept and y intercept respectively.
So converting equation (i):
$4x - 3y = 12$
Dividing 12 in LHS and RHS to get RHS as 1.
$
\Rightarrow \dfrac{{4x}}{{12}} - \dfrac{{3y}}{{12}} = \dfrac{{12}}{{12}} \\
\Rightarrow \dfrac{x}{3} - \dfrac{y}{4} = = 1 \\
\Rightarrow \dfrac{x}{3} + \dfrac{y}{{ - 4}} = 1..........................\left( {iii} \right) \\
$
So now comparing (iii) and (ii) we can say that $a = 3\,{\text{and}}\,b = - 4$ such that:
\[
{\text{x intercept}} = \left( {3,0} \right) \\
{\text{y intercept}} = \left( {0, - 4} \right) \\
\]
Now plotting the graph using the results that we have found.
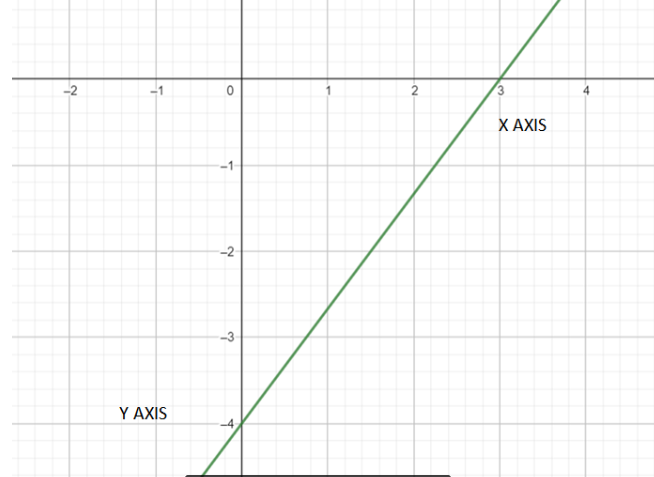
The above graph shows the plot of $4x - 3y = 12$.
Note: While approaching similar graphical questions one should find as many points as possible from the given conditions and common knowledge. Also one must be careful while doing the solution. Also while plotting the graph one must choose appropriate scale considering the values that should be plotted such that our every value can be represented on the graph.
Complete step by step solution:
Given
$4x - 3y = 12..........................\left( i \right)$
For graphing the given equation we need to find the x intercept and y intercept by using the
formula $\dfrac{x}{a} + \dfrac{y}{b} = 1$.
x intercept is the point where the graph touches X axis such that y=0 and y intercept is the point where the graph touches the Y axis such that x=0 .
So we know:
$\dfrac{x}{a} + \dfrac{y}{b} = 1...........................\left( {ii} \right)$
So we need to convert equation (i) in terms of (ii) and thereby find the values of
$a\,{\text{and}}\,b$ which are x intercept and y intercept respectively.
So converting equation (i):
$4x - 3y = 12$
Dividing 12 in LHS and RHS to get RHS as 1.
$
\Rightarrow \dfrac{{4x}}{{12}} - \dfrac{{3y}}{{12}} = \dfrac{{12}}{{12}} \\
\Rightarrow \dfrac{x}{3} - \dfrac{y}{4} = = 1 \\
\Rightarrow \dfrac{x}{3} + \dfrac{y}{{ - 4}} = 1..........................\left( {iii} \right) \\
$
So now comparing (iii) and (ii) we can say that $a = 3\,{\text{and}}\,b = - 4$ such that:
\[
{\text{x intercept}} = \left( {3,0} \right) \\
{\text{y intercept}} = \left( {0, - 4} \right) \\
\]
Now plotting the graph using the results that we have found.
The above graph shows the plot of $4x - 3y = 12$.
Note: While approaching similar graphical questions one should find as many points as possible from the given conditions and common knowledge. Also one must be careful while doing the solution. Also while plotting the graph one must choose appropriate scale considering the values that should be plotted such that our every value can be represented on the graph.