
How do you graph the inequality x < 1 on a coordinate plane?
Answer
456.3k+ views
Hint: The plot of an inequality is an area on a coordinate plane. First, plot the line for the equation
Complete step by step answer:
Let us first understand what is meant by an inequality.
As the same suggests, inequality refers to something (say some variable) being not exactly equal to something. In other words, we can say that the value of the quantity is not exactly known but we do know some restriction or condition for the value of the quantity.
For example, suppose we have a stick in our hand and we want to measure the length of that stick. However, we do not have any measuring instrument to measure its length. Now, we approximate the length of the stick and we surely know that the stick cannot be more than one metre (by using some other stick whose length is exactly one metre).
Here, the length (L) of the stick is unknown but it is true that the length is less than one metre.
i.e. L < 1m
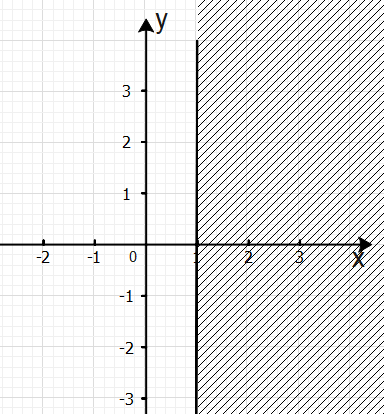
It is given that x < 1.
Now, if we plot the above inequality then we have to consider all the points on the coordinate plane where the x-coordinate is greater than one. It will be an area where all the points have x < 1.
We can shade that area by drawing the line
We know that line
Note: Note that the first step for plotting the region where the inequality holds true, is to write and the plot the equation by replacing the inequality by equal sign.
If the plot is of a line, then the area above the line is for the inequality which has greater than sign and the area below the line is for lesser than sign.
Complete step by step answer:
Let us first understand what is meant by an inequality.
As the same suggests, inequality refers to something (say some variable) being not exactly equal to something. In other words, we can say that the value of the quantity is not exactly known but we do know some restriction or condition for the value of the quantity.
For example, suppose we have a stick in our hand and we want to measure the length of that stick. However, we do not have any measuring instrument to measure its length. Now, we approximate the length of the stick and we surely know that the stick cannot be more than one metre (by using some other stick whose length is exactly one metre).
Here, the length (L) of the stick is unknown but it is true that the length is less than one metre.
i.e. L < 1m
It is given that x < 1.
Now, if we plot the above inequality then we have to consider all the points on the coordinate plane where the x-coordinate is greater than one. It will be an area where all the points have x < 1.
We can shade that area by drawing the line
We know that line

Note: Note that the first step for plotting the region where the inequality holds true, is to write and the plot the equation by replacing the inequality by equal sign.
If the plot is of a line, then the area above the line is for the inequality which has greater than sign and the area below the line is for lesser than sign.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it




