
What is the greatest common factor of
Answer
430.8k+ views
Hint: Greatest common factor also called GCF. The greatest common factor of two or more numbers is the largest possible number which divides the given numbers without any remainder.
We can find GCF by any of the following methods
1. Prime factorization method.
2. Listing the common factors method.
3. Long division method.
Complete step-by-step answer:
From the question it is clear that we have to find the greatest common factor of
From the basic concepts of mathematics, we know that the greatest common factor of two or more numbers is the largest possible number which divides the given numbers without any remainder.
Now let us try to find the greatest common factor of
In the prime factorization method, first we have to write the given numbers as the product of prime numbers.
Now, Let us try to write
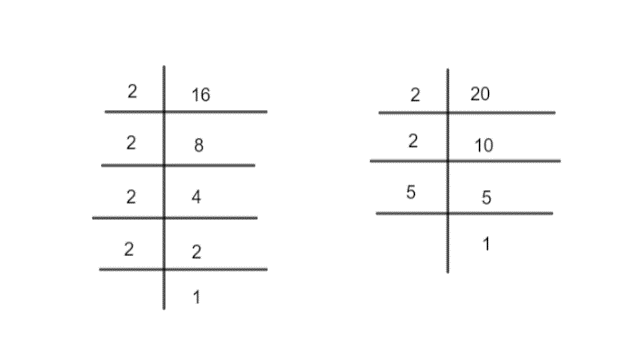
Prime factorization of
Prime factorization of
From the prime factorization of
The greatest common factor is the product of factors that are common to each of the given numbers.
so GCF is
Now we can conclude that the greatest common factor of
Note: While doing factorization we have to take only factors that are prime numbers. to make this method simple try to factorize the given numbers in the pictorial manner as shown. Students should be careful while doing calculations because it may lead to big errors in the answer.
We can find GCF by any of the following methods
1. Prime factorization method.
2. Listing the common factors method.
3. Long division method.
Complete step-by-step answer:
From the question it is clear that we have to find the greatest common factor of
From the basic concepts of mathematics, we know that the greatest common factor of two or more numbers is the largest possible number which divides the given numbers without any remainder.
Now let us try to find the greatest common factor of
In the prime factorization method, first we have to write the given numbers as the product of prime numbers.
Now, Let us try to write

Prime factorization of
Prime factorization of
From the prime factorization of
The greatest common factor is the product of factors that are common to each of the given numbers.
so GCF is
Now we can conclude that the greatest common factor of
Note: While doing factorization we have to take only factors that are prime numbers. to make this method simple try to factorize the given numbers in the pictorial manner as shown. Students should be careful while doing calculations because it may lead to big errors in the answer.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

What does R mean in math class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





