
How do you graph
Answer
468.9k+ views
Hint: Here we have to plot a graph. In this given equation by giving the values to the x like 0, 1, 2, 3, … simultaneously we get the values of y with respect to the x value. After getting the x and y values, write the coordinates of the given equation in the form of (x,y), by using the coordinates construct the required graph of the given equation.
Complete step-by-step answer:
Given equation in the form of linear equation in the form of two variables x and y
Consider the equation
Now, by giving the x values … -3, -2, -1, 0, 1, 2, 3, … to the above equation simultaneously we get the values of y
When we substitute the value of x=-3, then
Therefore, co-ordinate
When we substitute the value of x=-2
Therefore, co-ordinate
When we substitute the value of x=-1
Therefore, co-ordinate
When we substitute the value of x=0
Therefore, co-ordinate
When we substitute the value of x=1
Therefore, co-ordinate
When we substitute the value of x=2
Therefore, co-ordinate
When we substitute the value of x=3, then
Therefore, co-ordinate
And so on …
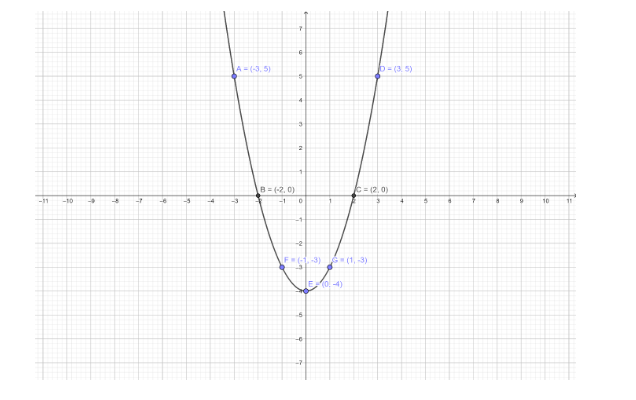
Hence by substituting the value of x we have determined some of the values or points we use to plot the graph.
The coordinates can be written in table as :
Hence, the graph of the given linear equation
Note: The question belongs to the concept of graph. By comparing the given equation to the equation of a line we calculate the slope and intercept. Or by choosing the value of x we can determine the value of y and then plotting the graphs for these points we obtain the result.
Complete step-by-step answer:
Given equation in the form of linear equation in the form of two variables x and y
Consider the equation
Now, by giving the x values … -3, -2, -1, 0, 1, 2, 3, … to the above equation simultaneously we get the values of y
When we substitute the value of x=-3, then
Therefore, co-ordinate
When we substitute the value of x=-2
Therefore, co-ordinate
When we substitute the value of x=-1
Therefore, co-ordinate
When we substitute the value of x=0
Therefore, co-ordinate
When we substitute the value of x=1
Therefore, co-ordinate
When we substitute the value of x=2
Therefore, co-ordinate
When we substitute the value of x=3, then
Therefore, co-ordinate
And so on …
Hence by substituting the value of x we have determined some of the values or points we use to plot the graph.
The coordinates can be written in table as :
| | | | | | | | |
| | | | | | | | |
| | | | | | | | |
Hence, the graph of the given linear equation

Note: The question belongs to the concept of graph. By comparing the given equation to the equation of a line we calculate the slope and intercept. Or by choosing the value of x we can determine the value of y and then plotting the graphs for these points we obtain the result.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Difference Between Plant Cell and Animal Cell

Given that HCF 306 657 9 find the LCM 306 657 class 9 maths CBSE

The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

What is pollution? How many types of pollution? Define it





